Selected Examples
Table of Contents
- Spatial Geometry
- The Quadratic Pyramid
- Analytic Geometry
- Intersection of Three Planes
- Interrsection of Line and Plane
- Conics
- Conics - Proof by Dandelin
- Instrument for constructing a parabola
- Differential Calculus
- Difference- and differential quotient
- Juice box
- Integral Calculus
- Dome of Speyer Cathedral
- Solid of Revolution
- Volume of Solids of Revolution
- Statistics
- Boxplot
- Probability
- Geometric Probabilty
- Descriptive Geometry
- Central projection of a Cube - Perspective
- Projection of a Sphere - Central Projection
- Physics
- Oszillating Pendulum and Lissajous-Figures
The Quadratic Pyramid
You see a quadratic pyramid.[br][br][b]Exercise[/b][br]Move point S and change the height of the pyramid.[br]Move the slider beneath.[br]What kind of triangles form the net of the pyramid?[br]Do you find any connection between the segment h, [math]h_a[/math] and [math]\frac{a}{2}[/math] in the shown triangle?
Andreas Lindner
Intersection of Three Planes
The following three equations define three planes:[br][math] \epsilon_{1}: a_{1} \cdot x + b_{1} \cdot y + c_{1} \cdot z = d_{1} [/math][br][math] \epsilon_{1}: a_{2} \cdot x + b_{2} \cdot y + c_{2} \cdot z = d_{2} [/math][br][math] \epsilon_{1}: a_{3} \cdot x + b_{3} \cdot y + c_{3} \cdot z = d_{3} [/math][br][br][br][b]Exercise[/b][br]a) Vary the sliders for the coefficient of the equations and watch the consequences.[br]b) Adjust the sliders for the coefficients so that[br][list][br][*] two planes are parallel,[br][*] three planes are parallel,[br][*] all three planes form a cluster of planes intersecting in one common line.[br][/list]
Conics - Proof by Dandelin
Intersection of plane and cone - proof by Dandelin[br][br][b]Exercise[/b][br]Move the yellow point on the generatrix in the upper part of the cone to modify it.[br]Move the red point in the blue plane to change the postion of this plane.[br]Subsequently slide the blue point on the lower circle.
Andreas Lindner
Difference- and differential quotient
In this applet you can see the function f: R → R; f(x) = 0,1·x² + 2[br][br]Move point B using the slider for Δx towards point A.[br]Take a note for the slope k of the secant through point A and B for Δx = 3,5 ; 3,0 ; 2,5; 2,0; 1,5; 1,2 and 1,1.[br]Which value could be assumed for the slope of the tangent in point A as a limit of the slopes for the secants?[br][br]Repeat the exercise for function f(x) = 0,1·x².
Andreas Lindner
Dome of Speyer Cathedral
The worksheet shows an exemplary account of the dome of the cathedral in Speyer, Germany.[br][br][b]Exercise[/b][br]Change your position with right mouse button and view the construction from different sides.[br]Show the prisms.[br]Vary the number n of prisms und watch the approximation of the sum of the volumes to a certain value.
Andreas Lindner
Boxplot
Visualization of a boxplot[br][br][b]Exercise[/b][br]Move different points which represent the values [math]a_1[/math] bis [math]a_{10}[/math] and watch the changing in the boxplot.
Andreas Lindner
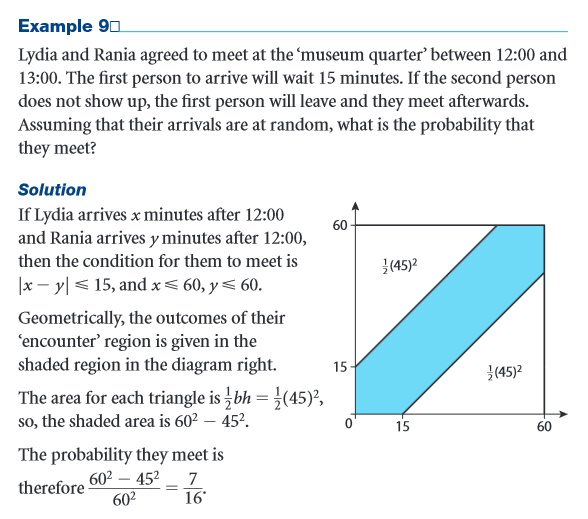
Geometric Probabilty
Geometric Probability
An example for geometric probabilty taken from Wazir & Garry: Standard Level Mathematics for the IB Diploma. 2014
Central projection of a Cube - Perspective
[b]Exercise[/b][br]Change your position with right mouse button and view the construction from different sides.[br][list][br][*]Move the position of the [color=#1551b5]center of the projection[/color] in horizontal and vertical direction.[br][*]Show the horizontal plane and auxiliary lines by clicking on the check boxes.[br][*]Enlarge oder shorten the sphere by moving point B.[br][*]Choose[i] front elevation[/i] (upright projection) in the style bar.[br][*]The position of the image plane can moved with point P.[br][/list]
Andreas Lindner
Oszillating Pendulum and Lissajous-Figures
A pendulum oszillating in two directions at the same time is performing a Lissajou figure.[br][br][b]Exercise[/b][br]Modify the amplitude and the frequency of the oszillations in both directions.[br][br][i]Hint: [br]The representation of the pendulum is exemplary. In reality the form of the suspension must be different and the length of the pendulum must be changed to achieve other frequencies of the pendulum.[/i]
Andreas Lindner