0

Augmented Reality: Ideas for Student Explorations

Tim Brzezinski, May 24, 2018

This GeoGebra book contains some lesson ideas for active, student-centered learning within GeoGebra's Augmented Reality app. This volume will continue to grow over time.

Getting Started with AR

Welcome to GeoGebra Augmented Reality!

1. Getting Started with GeoGebra Augmented Reality

Getting Started with GeoGebra Augmented Reality

Quick (Silent) Demo

Getting Started:

Be sure to download GeoGebra Augmented Reality on your device first. [br][br][url=https://itunes.apple.com/app/geogebra-augmented-reality/id1276964610&utm_source=Download+page&utm_medium=Website&utm_campaign=GeoGebra+Augmented+Reality+for+iOS][b][color=#1e84cc]Link to GeoGebra Augmented Reality app from App Store[/color][/b][/url][color=#1e84cc]. [br][/color]

Directions:

1) Open up [b][color=#1e84cc]GeoGebra Augmented Reality[/color][/b] app on iPad or iPhone. [br][br]2) Go to MENU. Select "[b]Two Functions[/b]". [br][br]3) Delete the first equation (provided). For blue (top) equation, enter [math]z=\sqrt{9-x^2-y^2}[/math]. For bottom equation, type in [math]z=-\sqrt{9-x^2-y^2}[/math]. [br][br]4) Take a ball (sphere) and show how this is a perfect model for such a sphere. [color=#ff0000]See 0:12 - 0:15 in video.[/color] [br][br]5) Delete bottom equation (pink). Change top (blue) equation to [math]z=\sqrt{4x^2+4y^2}[/math]. (See 0:30 in video). This creates a cone. [color=#ff0000]If you have a funnel, you can show what you see in video from 0:44 to 0:54. [/color][br][br]6) Enter new pink equation z = 3. This illustrates one of the four main conic section types: A CIRCLE. [color=#ff0000]See 1:02 to 1:13 in video.[/color] [br][br]7) Now change pink equation to z = 2x + 1. This will create a cross section (intersection) that is a PARABOLA (2nd type of conic section). [color=#ff0000]See 1:16 - 1:31 in video. [br][/color][br]8) Now change pink equation to z = 0.5x + 3. This will create a cross section (intersection) that is an ELLIPSE (3rd type of conic section). [color=#ff0000]See 1:32 to 1:44 in video. [/color][br][br]9) Now change pink equation to z = 5x + 2. This will create a cross section (intersection) that is a HYPERBOLA (4th type of conic section). [color=#ff0000]See 1:45 to END in video. [/color][br][br]

2.

Ideas for Student Activities & Explorations

New to GeoGebra AR? Check out these lesson ideas and explorations!

1. Conic Section Explorations
2. Exploring Function Transformations in 3D
3. Rotating about X-AXIS: Creating Surfaces of Revolution in GGB AR
4. Rotating about Y-AXIS: Creating Surfaces of Revolution in GGB AR
5. Gabriel's Horn: Virtual Exploration in GeoGebra Augmented Reality
6. How to Create Surfaces of Revolution in GeoGebra Augmented Reality

2.1.

Conic Section Explorations

Before we explore conic section types in GeoGebra Augmented Reality, let's explore them first in GeoGebra 3D Graphing Calculator app!

1.

[b][color=#ff00ff]Note the equation of the plane is z = some constant. [br][br][/color][/b][color=#ff00ff]Change the equation of this pink plane to z = 2. Then change it to z = 1. Then change it to z = 4. [/color][br][br]How would you describe the intersection of this [b][color=#ff00ff]plane[/color][/b] and [b][color=#1e84cc]cone[/color][/b]?

2.

Change the [b][color=#ff00ff]equation of the plane[/color][/b] to [math]z=x+1[/math]. How would you describe the intersection of this [b][color=#ff00ff]plane[/color][/b] and [b][color=#1e84cc]cone[/color][/b] now?

3.

Now change the [b]equation of the plane [/b]to [math]z=0.5x+2.5[/math]. How would you describe the intersection of this [b][color=#ff00ff]plane[/color][/b] and [b][color=#1e84cc]cone[/color][/b] now?

4.

Change the [b][color=#ff00ff]equation of the plane[/color][/b] to [math]z=3x+1.5[/math]. How would you describe the intersection of this [b][color=#ff00ff]plane[/color][/b] and [b][color=#1e84cc]cone[/color][/b] now?

Now let's explore these conic sections in GeoGebra Augmented Reality! (Silent "how-to" screencast)

Also: Quick Summary of All Conic Section Types & How They're Formed

2.2.

2.3.

2.4.

2.5.

2.6.

3.

AR Modeling Tips & Hints

This chapter contains some hints for students to help complete some of the challenges in the previous chapter (AR Modeling Challenges).

1. From 2D to 3D Modeling: It's Easier Than You Think!
2. How Domain Restrictions Can Help Students Model in GeoGebra Augmented Reality
3. Creating Duplicate Objects in 3-Space
4. Hint for Some GeoGebra AR Modeling Challenges
5. Quick AR Illustration: Projection of a Surface onto the xy-Plane
6. Square Pyramid with Hearts: GeoGebra Augmented Reality Demo

3.1.

From 2D to 3D Modeling: It's Easier Than You Think!

Math Teachers:

For students that have studied various classes of functions (linear, quadratic, square root, trigonometric), [b][color=#1e84cc]YOU CAN engage them in 3D modeling activities [/color][/b](& challenges) within GeoGebra Augmented Reality! [br][br]In this screencast below, note the two surface equations[br][math]z=\sqrt{16-x^2}[/math] and [math]z=-\sqrt{16-x^2}[/math]. If we were to replace [i]z[/i] with [i]y, [/i]we would have the equations of the top and bottom halves (respectively) of 2 semicircles with radius = 4 units we would typically have students graph in the coordinate plane. [br][br]Yet instead - here, we write [i]z[/i] as a function of [i]x [/i]and restrict the domain of this surface to be [math]\left|y\right|\le0.876[/math], or [math]-0.876\le y\le0.876[/math]. [br][br]More info can be found below the screencast. [br][br]

Quick (Silent) Demo

[b][color=#ff00ff]Yet where did this domain restriction come from? [/color] [/b]That part is easy. Before building the model, we measured the radius of this cylindrical coffee maker. Here, [i]r[/i] = 10.5 cm and the height of this cylinder = 4.6 cm. Since we chose 4 UNITS in GeoGebra Augmented Reality to represent 10.5 cm = radius, we need to determine how many units represent the height of this cylinder. [br][br]Thus, [math]\frac{4u}{10.5cm}=\frac{?u}{4.6cm}[/math]. And upon solving, we get ? = 1.75 units. Since we chose the plane [i]y[/i] = 0 to split this cylindrical lateral area in half, this surface needs to extend 1.75u / 2 = 0.876 units in both the positive y-direction and negative y-direction. Hence, the need for the domain restriction [math]\left|y\right|\le0.876[/math]. [br][br]And this simple level of proportional reasoning is a task that MANY STUDENTS can do by the time they study various classes of functions in high school. [br][br][color=#1e84cc][i]Building 3D mathematical models of real-world objects IS a task MANY STUDENTS CAN DO after studying various classes of functions. So why restrict modeling to only be within the (2D) coordinate plane? [/i][/color]

3.2.

3.3.

3.4.

3.5.

3.6.

4.

Modeling Challenges (Easier)

1. Fence Post with Square Cross Sections (AR Modeling Challenge)
2. Square Prism with Holes (AR Modeling Challenge)
3. Toblerone Candy (AR Modeling Challenge)
4. Popcorn Bowl (AR Modeling Challenge)
5. Halogen Lamp (AR Modeling Challenge)
6. Holiday Bulb (AR Modeling Challenge)
7. Deodorant Cap (AR Modeling Challenge)
8. Square Pyramid with Holes (AR Modeling Challenge)
9. Trash Can Cover (AR Modeling Challenge)
10. Bowling Ball Reconstruction (AR Modeling Challenge)
11. Traffic Cone (AR Modeling Challenge)
12. Reading Lampshade (AR Modeling Challenge)
13. Lampshade 1 (AR Modeling Challenge)
14. Lampshade 2 (AR Modeling Challenge)
15. Light Fixture (AR Modeling Challenge)
16. Mailbox Top (AR Modeling Challenge)
17. Airport Hangar (AR Modeling Challenge)
18. Headphones Case (AR Modeling Challenge)
19. Igloo (AR Modeling Challenge)

4.1.

Fence Post with Square Cross Sections (AR Modeling Challenge)

Students:

Using only 2 surface equations with domain restrictions, try to reconstruct this piece of wooden fence within GeoGebra Augmented Reality. [br][br][b]Modeling Clue:[/b][br][br]Cross sections of any plane parallel to the parallel to the plane y = 0 are squares whose diagonals are each 6 units long. [br][br][b]Hint: [br][/b]What kind of 2D function has a graph that comes in the shape of the letter "V"?

Quick Demo

4.2.

4.3.

4.4.

4.5.

4.6.

4.7.

4.8.

4.9.

4.10.

4.11.

4.12.

4.13.

4.14.

4.15.

4.16.

4.17.

4.18.

4.19.

5.

Modeling Challenges (Harder)

1. Ice Cream Cone Head (AR Modeling Challenge)
2. Muffin Tin with Muffin (AR Modeling Challenge)
3. Random 3D Solid (AR Modeling Challenge)
4. Two Pieces of a Fence (AR Modeling Challenge)
5. Hotel Lounge Table (AR Modeling Challenge)
6. Bike Rack (AR Modeling Challenge)
7. Two Spheres (AR Modeling Challenge)
8. Four Spheres (AR Modeling Challenge)
9. Restaurant Light (AR Modeling Challenge)
10. Planter (AR Modeling Challenge)
11. Pyramid Section (AR Modeling Challenge)
12. Regular Octahedron (AR Modeling Challenge)
13. Rotated Cylinder (AR Modeling Challenge)
14. Intersecting Cylinders with Hollow Middle (AR Modeling Challenge)
15. Wiffle Ball (AR Modeling Challenge)

5.1.

Ice Cream Cone Head (AR Modeling Challenge)

Can you author 2 surface equations (with possible domain restrictions) to reconstruct this 2-faced virtual ice cream cone within GeoGebra Augmented Reality? [br][br][b]Clues:[br][br][/b]1) The blue surface is a hemisphere with radius = 3. [br][br]2) The top point of the hemisphere is (0, 0, 5).[br][br]3) The apex of the cone is at (0,0,5).[br][br]4) The projection of all 4 eyes onto the plane z = 0 are circles with centers [math]\left(\pm0.8,\pm1.5\right)[/math] and [math]r=\frac{\sqrt{10}}{10}[/math]. [br][br]5) The projection of the one mouth boundary onto the plane z = 0 has equation [math]y=0.09x^2-2.8[/math]. [br][br]6) The projection of the other mouth boundary onto the plane z = 0 has equation [math]y=-0.09x^2+2.8[/math]. [br][br][b]For additional challenges, see the text below this screencast. [/b]

Demo

Additional Challenges:

1) Reconstruct this same surface, yet reflect it about the plane z = 0. [br] I.E: "Flip it upside down"! [br][br]2) Reconstruct this same surface, yet put 2 additional mouths on here that are the mouths seen here, yet [br] rotated [math]90^\circ[/math] about (0,0,0).

5.2.

5.3.

5.4.

5.5.

5.6.

5.7.

5.8.

5.9.

5.10.

5.11.

5.12.

5.13.

5.14.

5.15.

6.

AR Modeling Demonstrations

With GeoGebra AR, the possibilities are seemingly ENDLESS! Here are a few illustrations of every-day, real-world, 3D objects that can be modeled effectively within GeoGebra AR. Enjoy!

6.1.

Quick Modeling of a Bench Within GeoGebra AR

Here, we quickly model a bench within GeoGebra Augmented Reality. [br][br]Note how changing the amplitude of this sinusoidal surface helps us quickly achieve building a better virtual model of this bench.

Quick Demo

6.2.

6.3.

6.4.

6.5.

6.6.

6.7.

6.8.

6.9.

6.10.

6.11.

6.12.

6.13.

6.14.

6.15.

6.16.

6.17.

6.18.

6.19.

6.20.

6.21.

6.22.

6.23.

6.24.

6.25.

6.26.

6.27.

6.28.

6.29.

6.30.

6.31.

6.32.

6.33.

6.34.

6.35.

6.36.

6.37.

6.38.

6.39.

6.40.

6.41.

6.42.

6.43.

6.44.

6.45.

6.46.

7.

Quick Illustrations

The first resource contains AR photos that Math Teachers have posted on Twitter. The remaining resources (displayed here using GeoGebra's 3D Graphing Calculator) are illustrations of other built-in solids that can be explored within GeoGebra's AR app.

1. Modeling with GeoGebra Augmented Reality: Project Ideas
2. Basic solids
3. Penrose triangle
4. Sierpinski pyramid
5. Football
6. Klein Bottle
7. Ruled Surface

7.1.

Modeling with GeoGebra Augmented Reality: Project Ideas

MATH TEACHERS:

We live in a 3-dimensional world! So why restrict students' creativity to being in the (2D) coordinate plane? [br][br]We can create 3D models of real-world objects within [url=https://www.geogebra.org/3d]GeoGebra's 3D Graphing Calculator.[/url][br]We can also use [b]GeoGebra Augmented Reality[/b] to virtually create & explore such surfaces! [br][br]Below are some photos found on Twitter. We'd love to add YOURS to the list too! [br][br][b][color=#1e84cc]Can you author surface equation(s) (of the form [/color][/b][math]z=[/math][b][color=#1e84cc] )[/color][/b] [b][color=#ff00ff]that model each of the items shown below? [/color][/b] [br][br][b]What other solids exist for which we can create 3D mathematical models? [/b]

Bird Bath (Photo Credit: Jose Luis Munoz)

[url=https://twitter.com/jlmunoz161/status/981103423813832704]Source[/url]

Easter Egg: GREAT Background Sign! (Photo Credit: Owen Elton)

[url=https://twitter.com/owenelton/status/980099069417869314]Source[/url]

Donuts! Hungry yet? (Photo Credit: Steve Phelps)

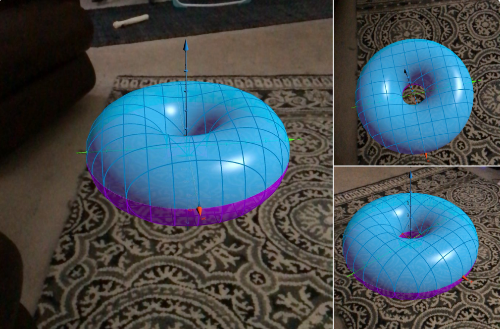
[url=https://twitter.com/giohio/status/1000036148390047744]Source[/url]

Pringles Potato Chip

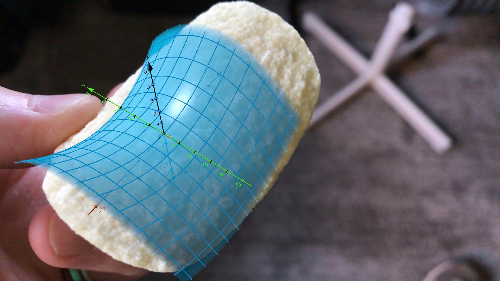
[url=https://twitter.com/dynamic_math/status/987171879143333889]Source[/url]

Conical Cup (Photo Credit: Steve Phelps)

[url=https://twitter.com/giohio/status/994268624721469440]Source[/url]

Toblerone Candy

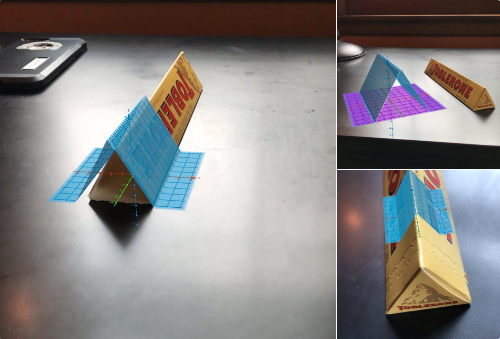
[url=https://twitter.com/dynamic_math/status/994725264956559360]Source[/url]

Roll of Paper Towels (Photo Credit: Steve Phelps)

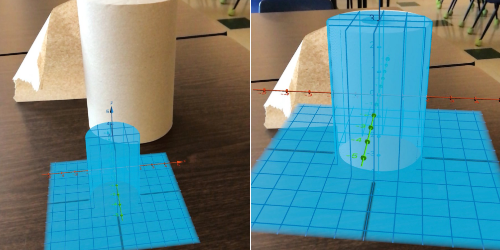
[url=https://twitter.com/giohio/status/994551940225871873]Source[/url]

Stairs (Photo Credit: Steve Phelps)

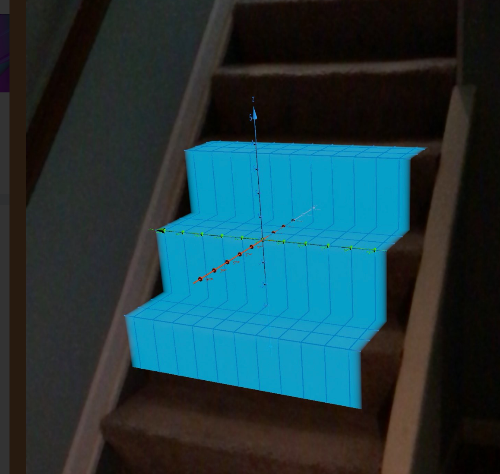
[url=https://twitter.com/giohio/status/1000064066096979970]Source[/url]

Hyperboloid of 1 Sheet Lattice (Photo Credit: Steve Phelps)

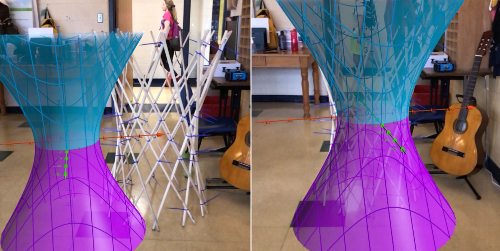
[url=https://twitter.com/giohio/status/994634527904616451]Source[/url]

Basket (Photo Credit: Steve Phelps)

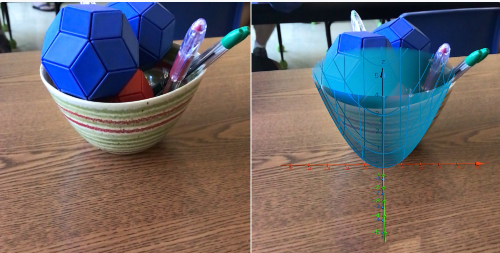
[url=https://twitter.com/giohio/status/994600185438396416]Source[/url]

SUBMIT YOUR GEOGEBRA AUGMENTED REALITY MODELING PHOTOS!

[b]Let's let this list of 3D project modeling ideas GROW! [/b][br][br]Submit your best photo(s) to dynamicmathsolutions@gmail.com [br][br]OR[br][br][b][color=#0000ff]If you're on Twitter,[/color][/b] post them there and be sure to tag [url=https://twitter.com/geogebra]@geogebra[/url], [url=https://twitter.com/dynamic_math]@dynamic_math[/url], #MTBoS, & #ITeachMath!

0

Augmented Reality: Ideas for Student Explorations

Table of Contents

Getting Started with AR

Getting Started with GeoGebra Augmented Reality

Quick (Silent) Demo

Getting Started:

Directions:

Ideas for Student Activities & Explorations

Conic Section Explorations

1.

2.

3.

4.

Now let's explore these conic sections in GeoGebra Augmented Reality! (Silent "how-to" screencast)

Also: Quick Summary of All Conic Section Types & How They're Formed

AR Modeling Tips & Hints

From 2D to 3D Modeling: It's Easier Than You Think!

Math Teachers:

Quick (Silent) Demo

Modeling Challenges (Easier)

Fence Post with Square Cross Sections (AR Modeling Challenge)

Students:

Quick Demo

Modeling Challenges (Harder)

Ice Cream Cone Head (AR Modeling Challenge)

Demo

Additional Challenges:

AR Modeling Demonstrations

Quick Modeling of a Bench Within GeoGebra AR

Quick Demo