Todo sobre FUNCIONES

Nerea Alemán Campoy, May 26, 2017

Si quieres saber qué es una función, cómo se representan, e.t.c... te aconsejo visualizar este libro.

Table of Contents

  1. ¿Qué es una función?
    1. Funciones elementales
  2. Funciones elementales
    1. Rectas
    2. Cuadrática
    3. Potenciales de exponente natural
    4. Potenciales de exponente entero negativo- racionales
    5. Logarítmicas
    6. Exponenciales
    7. Irracional
    8. Trigonométricas
  3. Función a trozos
    1. A trozos
  4. Operaciones con funciones
    1. Suma
    2. Resta
    3. Producto de un número real por una función
    4. Producto
    5. Cociente
  5. F.compuesta
    1. f o g
  6. F.inversa
    1. f^-1

1.

¿Qué es una función?

Una función (f) es una relación entre un conjunto dado X y otro conjunto de elementos Y de forma que a cada elemento x del le corresponde un único elemento f(x).

  • 1. Funciones elementales

1.1.

Funciones elementales

– Maria Carmen Prieto Jiménez

2.

Funciones elementales

Una función elemental es aquella que se puede construir a partir de una cantidad finita de exponenciales, logaritmos, constantes, una variable, raíces de ecuaciones mediante composición y combinaciones, mediante la utilización de las cuatro operaciones elementales que serían, suma, resta, multiplicación y división.

  • 1. Rectas
  • 2. Cuadrática
  • 3. Potenciales de exponente natural
  • 4. Potenciales de exponente entero negativo- racionales
  • 5. Logarítmicas
  • 6. Exponenciales
  • 7. Irracional
  • 8. Trigonométricas

2.1.

Rectas

Existen cuatro tipos de funciones cuya gráfica es una recta:[br][list=1][*]FUNCIÓN AFÍN: y=ax+b[/*][*]FUNCIÓN LINEAL: y=ax (pasa por el origen)[/*][*]FUNCIÓN CONSTANTE: Y=k[/*][*]FUNCIÓN CONSTANTE: x=k[/*][/list]Sólo hacen falta los puntos de corte con los ejes y tabla de valores.
– José Alberto Torres Ríos, Nerea Alemán Campoy

2.2.

–

2.3.

–

2.4.

–

2.5.

–

2.6.

–

2.7.

–

2.8.

–

3.

Función a trozos

  • 1. A trozos

3.1.

A trozos

Funciones definidas en intervalos.[br]TABLA DE VALORES Y SIEMPRE DAR LOS EXTREMOS (cerrados o abiertos)[br]Se ponen en común todas las funciones elementales existentes en un mismo plano.
– José Luis Muñoz Casado, Nerea Alemán Campoy

4.

Operaciones con funciones

  • 1. Suma
  • 2. Resta
  • 3. Producto de un número real por una función
  • 4. Producto
  • 5. Cociente

4.1.

Suma

Forma: (f+g)(x) =f(x) + g(x)[br]DOMINIO: [math]\forall[/math][math]\epsilon[/math] Dom f [math]\cap[/math] Dom g[br]
– Victor Manuel Uribe Villegas, Nerea Alemán Campoy

4.2.

–

4.3.

–

4.4.

–

4.5.

–

5.

F.compuesta

  • 1. f o g

5.1.

f o g

FORMA:[br] f g[br]R[math]\hookrightarrow[/math]R[math]\hookrightarrow[/math]R[br]x[math]\rightharpoondown[/math]f(x)[math]\rightharpoonup[/math] g(f(x))[br][br][br]f o g se lee: g compuesto de f y no son conmutativas (f o g[math]\ne[/math] g o f)
FORMA
– Nerea Alemán Campoy

6.

F.inversa

  • 1. f^-1

6.1.

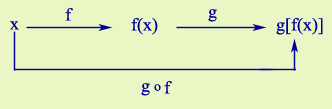
f^-1

Sustituimos la x por la y o viceversa y despejamos la y en función de x.[br][br]f o f ^-1= f^-1 o f= i(x) (es conmutativa solo en este caso)[br]Pasa por el origen y es el eje de simetría entre dos líneas.
F. inversa
F. identidad
– Nelson Lillo Terán ( Gaiano Andino ), valeria, Nerea Alemán Campoy

Information