Mitad del cubo (3)
De aspecto similar a mitad del cubo (2), en este caso los hexágonos que se forman tienen lados paralelos de distinta longitud, excepto para t=1/2 que también se obtiene un hexágono regular.[br]
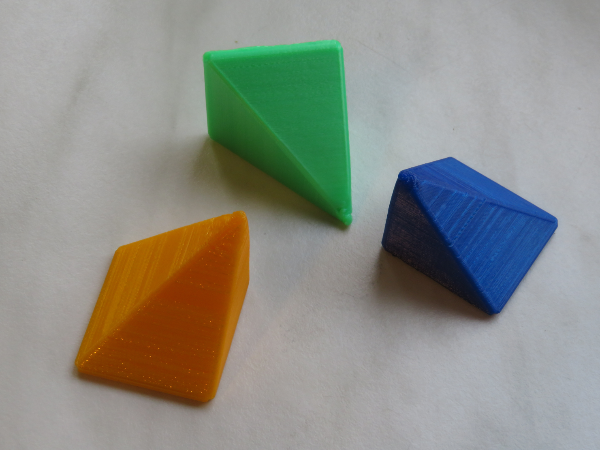
Dividing a cube by 3

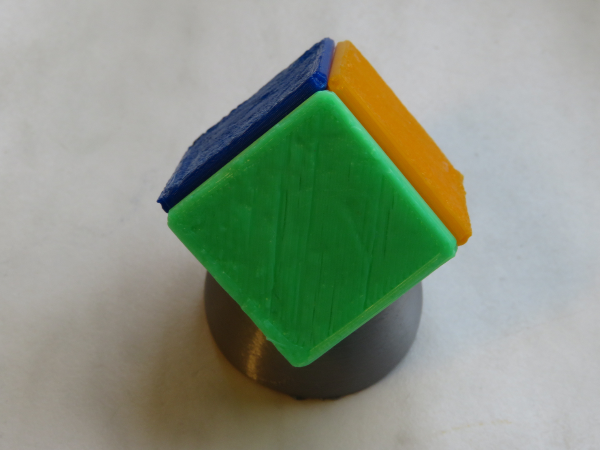
Dividing a cube by 4
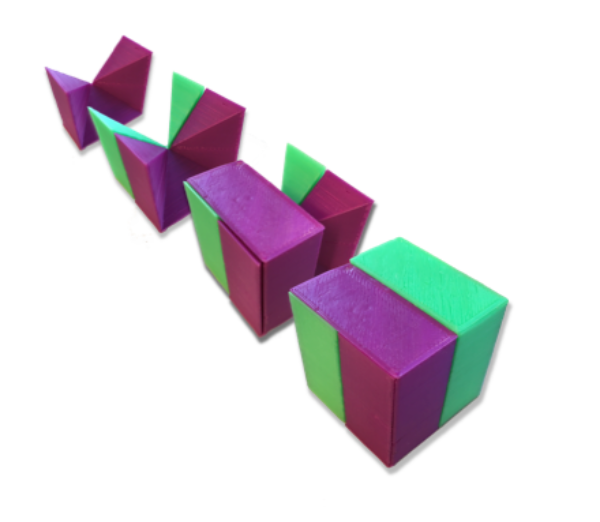
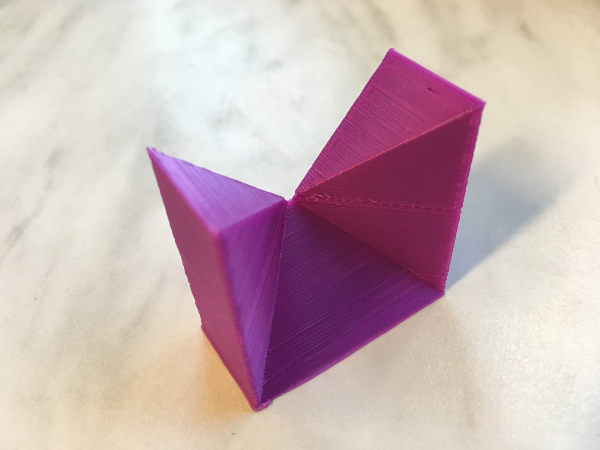
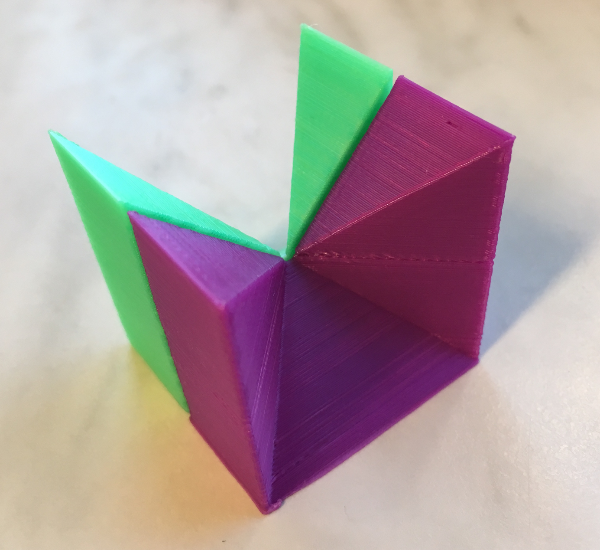
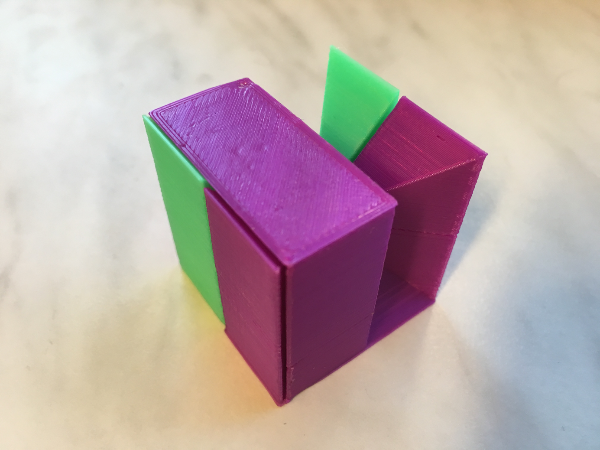
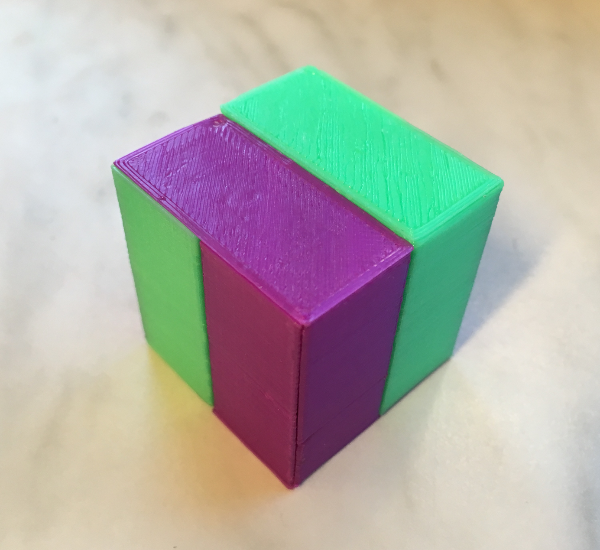
MMC exemplo2
Dividing a cube by 6
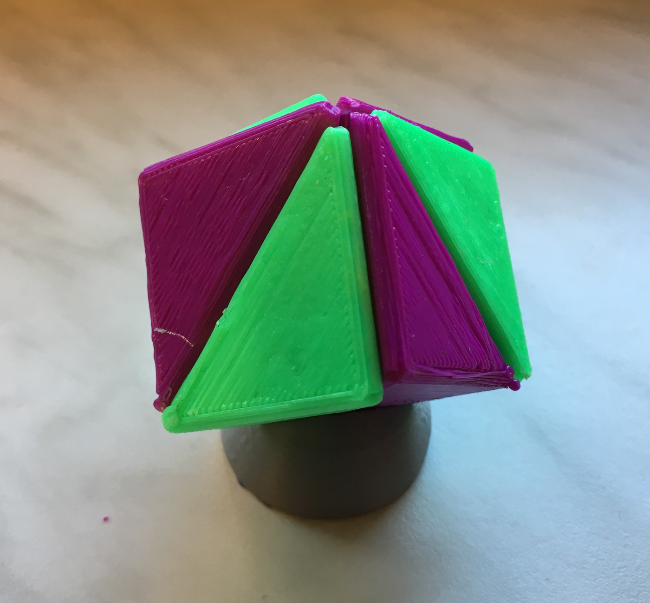
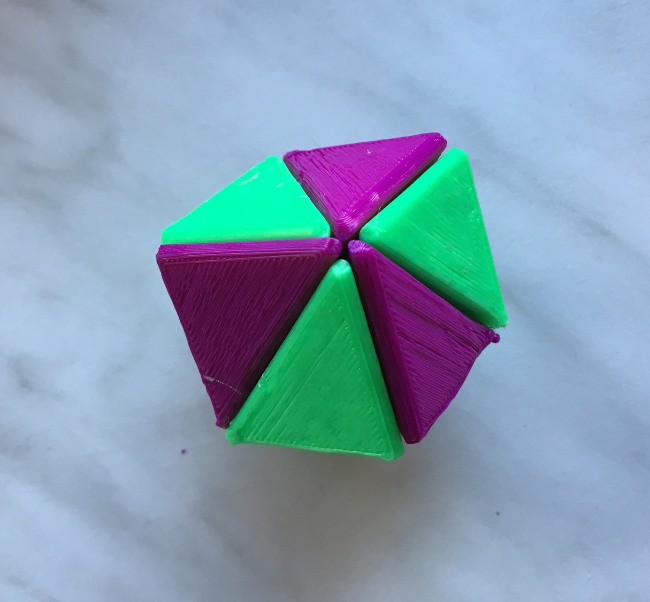

Dividing a cube by 7
[math]\frac{1}{7}+\left(\frac{1}{7}\right)^2+\left(\frac{1}{7}\right)^3+\left(\frac{1}{7}\right)^4+\left(\frac{1}{7}\right)^5+...=[/math]
Think how can you divide a cube into 7 equal parts, all of them with the same volume and surface as well.
1/8 cube
this version is able to download as STL
this version is NOT able to download as STL
Adapted from [url=http://gallery.bridgesmathart.org/exhibitions/2018-bridges-conference/shen-guan-shih]http://gallery.bridgesmathart.org/exhibitions/2018-bridges-conference/shen-guan-shih[/url].
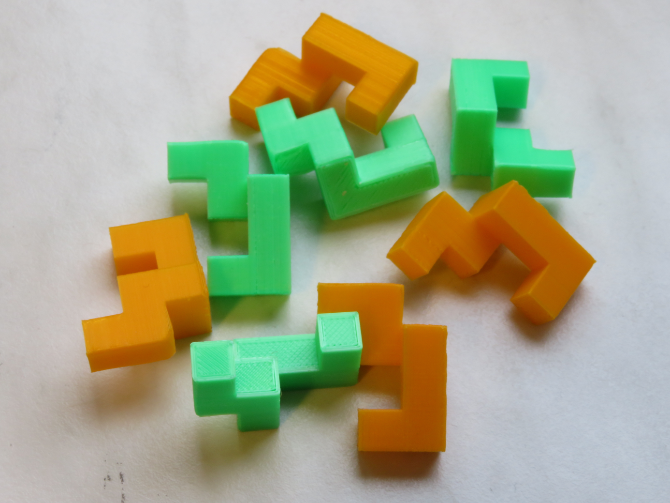
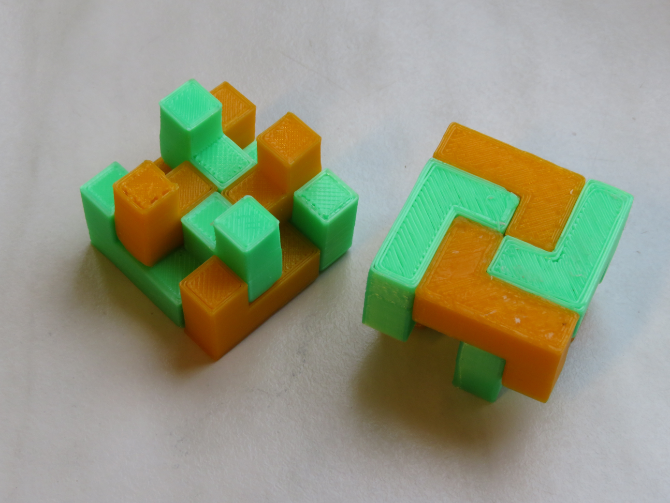
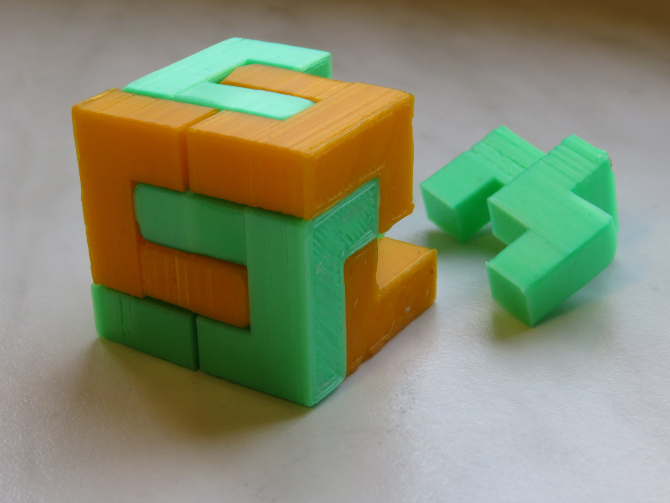
Dividing a cube by 9
reference: [url=https://www.quora.com/How-would-you-cut-a-cube-into-9-pieces-with-equal-volume-and-equal-surface-area]https://www.quora.com/How-would-you-cut-a-cube-into-9-pieces-with-equal-volume-and-equal-surface-area[/url]
12-Lizard

Inspirado no livro [url=https://www.amazon.com.br/Mind-Blowing-Paper-Puzzles-Ebook-Interlocking-ebook/dp/B07NQSLQBX]Mind-Blowing Paper Puzzles[/url], de Haruki Nakamura.
