UNIT 2 LINEAR FUNCTIONS

Rachel Turner, Sep 30, 2020

THIS UNIT EXPLORES THE LINEAR RELATIONSHIP BETWEEN 2 VARIABLES.

Table of Contents

  1. LESSON 1
    1. UNIT 2 LESSON 1 WARMUP
    2. UNIT 2 LESSON 1 RATE OF CHANGE
    3. UNIT 2 LESSON 1 LINEAR FUNCTIONS
  2. LESSON 2
    1. UNIT 2 LESSON 2 WARMUP
    2. UNIT 2 LESSON 2 SLOPE OF A LINE
    3. UNIT 2 LESSON 2 SLOPE FORMULA
    4. UNIT 2 LESSON 2 TYPES OF SLOPE
  3. LESSON 3
    1. UNIT 2 LESSON 3 WARMUP
    2. UNIT 2 LESSON 3 SLOPE - INTERCEPT FORM
    3. UNIT 2 LESSON 3 GRAPHING SLOPE - INTERCEPT FORM
    4. UNIT 2 LESSON 3 WRITING SLOPE - INTERCEPT FORM
    5. UNIT 2 LESSON 3 HOW SLOPE - INTERCEPT FORM CHANGES
  4. LESSON 4
    1. UNIT 2 LESSON 4 WARMUP
    2. UNIT 2 LESSON 4 - DEVELOPING POINT SLOPE FORM
    3. UNIT 2 LESSON 4 GRAPH A LINE IN POINT-SLOPE FORM
  5. LESSON 5
    1. UNIT 2 LESSON 5 WARMUP
    2. UNIT 2 LESSON 5 WRITING LINEAR EQUATIONS
    3. UNIT 2 LESSON 5 WRITING EQUATIONS PRACTICE

1.

LESSON 1

INTRODUCTION TO LINEAR FUNCTIONS [list] [*]CALCULATE THE RATE OF CHANGE OF A FUNCTION AND, IF CONSTANT, THE INITIAL VALUE OF THE FUNCTION [*]DETERMINE IF A RELATIONSHIP IS LINEAR BY ANALYZING THE RATE OF CHANGE [/list]

  • 1. UNIT 2 LESSON 1 WARMUP
  • 2. UNIT 2 LESSON 1 RATE OF CHANGE
  • 3. UNIT 2 LESSON 1 LINEAR FUNCTIONS

1.1.

UNIT 2 LESSON 1 WARMUP

Use the pen tool to draw a line to from the word to its matching definition. Click the check your answer at the bottom to check your answers. You do not need to type something in the text box if you drew lines.
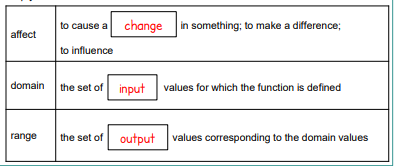
INDEPENDENT AND DEPENDENT VARIABLES
Michaela earns $8 per hour at an after-school job.[br]For a function that gives the amount earned for working a given amount of hours:[br][list][*]The independent variable (___________) is the number of hours workend.[/*][*]The dependent variable (output) is the ________________________.[/*][/list]
– Rachel Turner

1.2.

–

1.3.

–

2.

LESSON 2

Slope of a Line [list] [*]Determine the slope of a line from a graph, table of values, or ordered pairs. [*]Identify if the slope of a linear relationship is zero, positive, negative, or undefined. [*]Interpret slope in the context of real-world scenarios. [/list]

  • 1. UNIT 2 LESSON 2 WARMUP
  • 2. UNIT 2 LESSON 2 SLOPE OF A LINE
  • 3. UNIT 2 LESSON 2 SLOPE FORMULA
  • 4. UNIT 2 LESSON 2 TYPES OF SLOPE

2.1.

UNIT 2 LESSON 2 WARMUP

WORDS TO KNOW
Decide which word goes to the definitions below.[br][table][tr][td]zero slope[/td][td]rate of change[/td][td]positive slope[/td][td]slope[/td][td]undefined slope[/td][td]negative slope[/td][/tr][/table][table][tr][td]________[/td][td]in a relation, the relationship between the change in the dependent value and the change in the independent value.[/td][/tr][tr][td]________[/td][td]a slope that occurs when all the values of the independent variable are the same; the slope of a vertical line[/td][/tr][tr][td]________[/td][td]a slope that occurs when all the values of the dependent variable are the same; the slope of a horizontal line[/td][/tr][tr][td]________[/td][td]in a function, the ratio of the change in the dependent value with respect to the change in the independent value[/td][/tr][tr][td]________[/td][td]quality of a relation in which the dependent variable increases as the independent variable increases[/td][/tr][tr][td]________[/td][td]quality of a relation in which the dependent variable decreases as the independent variable increases[/td][/tr][/table]
RATE OF CHANGE: DEFINITION
The [b]rate of change[/b] of a function is the ____________ of the change in the dependent variable with respect to the change in the _____________ variable.[br][br]That is the same as [math]\frac{\Delta y}{\Delta x}[/math]
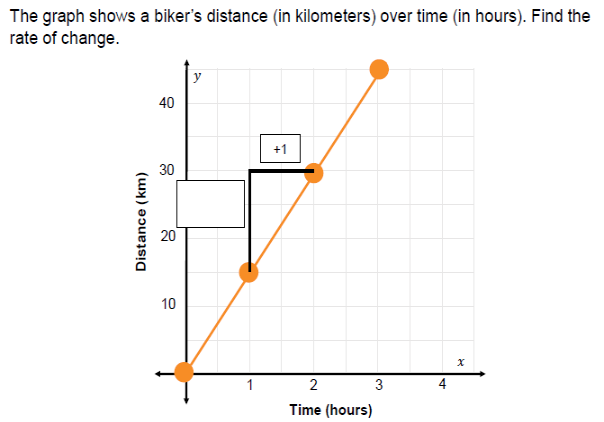
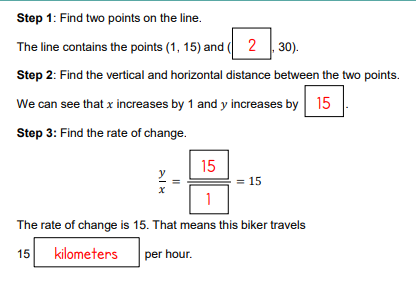
RATE OF CHANGE: GRAPHING
What is the vertical change of the graph above?
– Rachel Turner

2.2.

–

2.3.

–

2.4.

–

3.

LESSON 3

Slope-Intercept Form of a Line [list] [*]Analyze how a change in a parameter of a linear function affects its graph or the scenario it represents [*]Identify the slope and y-intercept of a linear function, and use them to graph the function [*]Write a linear function, in slope-intercept form, for a given relationship [/list]

  • 1. UNIT 2 LESSON 3 WARMUP
  • 2. UNIT 2 LESSON 3 SLOPE - INTERCEPT FORM
  • 3. UNIT 2 LESSON 3 GRAPHING SLOPE - INTERCEPT FORM
  • 4. UNIT 2 LESSON 3 WRITING SLOPE - INTERCEPT FORM
  • 5. UNIT 2 LESSON 3 HOW SLOPE - INTERCEPT FORM CHANGES

3.1.

UNIT 2 LESSON 3 WARMUP

WORDS TO KNOW
– Rachel Turner

3.2.

–

3.3.

–

3.4.

–

3.5.

–

4.

LESSON 4

POINT SLOPE FORM OF A LINE [list] [*]Graph a line given its equation in point-slope form, identifying the slope and intercepts [*]Write the equation of a line given its slope and a point on the line in point-slope form, and express the relationship as a function. [/list]

  • 1. UNIT 2 LESSON 4 WARMUP
  • 2. UNIT 2 LESSON 4 - DEVELOPING POINT SLOPE FORM
  • 3. UNIT 2 LESSON 4 GRAPH A LINE IN POINT-SLOPE FORM

4.1.

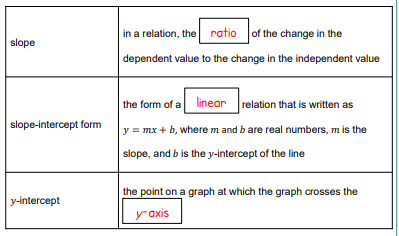
UNIT 2 LESSON 4 WARMUP

WORDS TO KNOW
[table][tr][td]REPRESENT[/td][td]to be an [color=#ff0000]example[/color] or expression of something[/td][/tr][tr][td]LINEAR FUNCTION[/td][td]a function that can be written in the form [color=#ff0000]y=mx+b[/color], where m and b are real numbers; consists of a set of ordered pairs all lying on the same line. [/td][/tr][tr][td]POINT-SLOPE FORM[/td][td]the form of a [color=#ff0000]linear[/color] relation that is [math]y-y_{_1}=m\left(x-x_{_1}\right)[/math] where m is the slope and [math]\left(x_{_1},y_{_1}\right)[/math] is a [color=#ff0000]point on the line[/color][/td][/tr][tr][td]SLOPE[/td][td]in a relation, the relationship between the [color=#ff0000]change[/color] in the dependent value and the change in the independent value[/td][/tr][/table]
WRITING A LINEAR FUNCTION
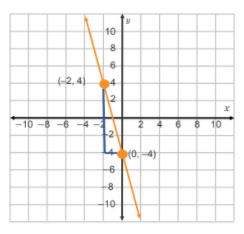
What is the slope of the line?
What is the y-intercept of the line?
What is the equation for the line?
– Rachel Turner

4.2.

–

4.3.

–

5.

LESSON 5

WRITING LINEAR EQUATIONS[list] [*]Use linear models to solve problems [*]Write two-variable linear equations in different forms using varying pieces of information about the relationships [/list]

  • 1. UNIT 2 LESSON 5 WARMUP
  • 2. UNIT 2 LESSON 5 WRITING LINEAR EQUATIONS
  • 3. UNIT 2 LESSON 5 WRITING EQUATIONS PRACTICE

5.1.

UNIT 2 LESSON 5 WARMUP

WORDS TO KNOW
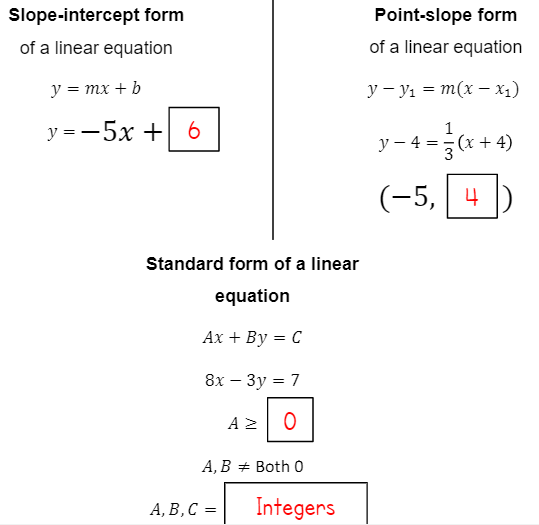
Match the terms to their definition:[br][table][tr][td][b]point-slope form[/b][/td][td][b]slope-intercept form[/b][/td][td][b]linear function[/b][/td][td][b]standard form [/b][/td][/tr][/table][br][table][tr][td]1. [b]_______[/b][/td][td][b][/b]the form [math]Ax+By=C[/math], where A, B and C are real numbers such that A and B are not both zero, A is positive if it is not zero, and the greatest common factor of A, B, and C is 1[/td][/tr][tr][td]2. _______[/td][td]a linear relation written as [math]y-y_{_1}=m\left(x-x_{_1}\right)[/math], where m is the slope and [math]\left(x_{_1},y_{_1}\right)[/math] is a point on the line[/td][/tr][tr][td]3. _______[/td][td][b][/b]a relationship between independent and dependent variables characterized by a constant rate of change, a highest degree of 1, and a graph that is a straight line[/td][/tr][tr][td]4. _______[/td][td]a linear relation written as [math]y=mx+b[/math], where m and b are real numbers, m is the slope and b is the y-intercept of the line[/td][/tr][/table]
FORMS OF LINEAR EQUATIONS
– Rachel Turner

5.2.

–

5.3.

–

Information