ellipse as a flat curve
locus
a locus is a geometric figure containing a set of points matching a specific condition.[br]For example: [br][list][*]the perpendicular bisecor of two points is the locus of the points at the same distance from two given points.[/*][*]the circle is the locus of twe points at the same distance from a given point.[br][/*][*]the bisector of two intersecting lines is the locus of the points at the sams distance from two given lines.[/*][/list]
ellipse as a locus
Een ellips is the locus of a point which moves in a plane such that the sum of its distances from two given points (called foci) add up to a constant.[br]This means you can draw an ellips with the help of a rope: Put down two stakes and loop a piee of rope around them. Pull the loop taut and mark the points aroud a curve.
applet
The method is illustrated in the applet below.[br][list][*]Drag the point F[sub]1[/sub] and define the distance between the two foci. [/*][*]Drag the point C and define the lenghts of the rope.[/*][*]Drag teh point Pand draw the ellipse.[/*][/list]
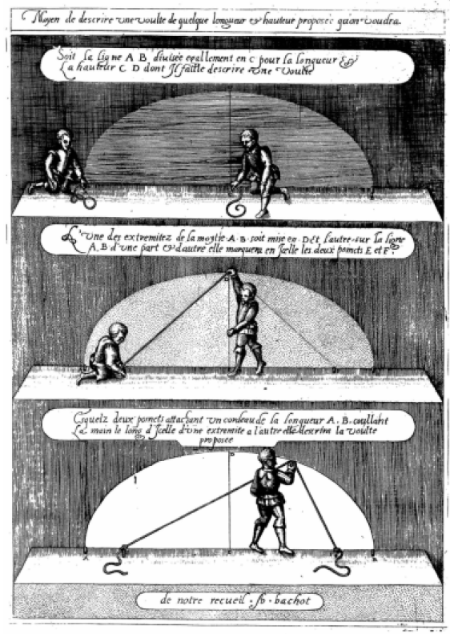
gardener's method
This construction is called [i]the gardener's method[/i] because it was used in Engelse gardens to mark flowerbeds. Architects and military ingeneers mention it in tractates.[br]E.g. Ambroise Bachot mentions the methode in his book from 1598 on architecture, fortifications and weapons of war.
