Construções Geométricas
[[b]Tradução [/b][i](em andamento)[/i][b] do livro "Geometric Constructions" de autoria de [url=https://www.geogebra.org/once_a_student] Ku, Yin Bon (Albert) [/url] [/b]] Esse é um curso interativo sobre [b][color=#1551b5] construções geométricas[/color][/b], um assunto fascinante que tem sido ignorado pela educação matemática comum. Ele é todo sobre construção de figuras geométricas usando ferramentas específicas como régua, compasso e outras. Esse tópico clássico da geometria é importante, porque: [list] [*] a base da geometria é em sua maioria inspirada pelo que podemos fazer com essas ferramentas de construção, e [*] envolve muita matemática elegante que mostra a interação entre geometria e álgebra. [/list] Há inúmeros exercícios de construção nesse curso. [color=#c51414] Ao construir com sucesso a(s) figuras(s) geométrica(s) requerida(s) em cada exercício, a mensagem "[b]Well done![/b]" irá aparecer.[/color] [b][color=#c51414]Pré-requisitos para esse curso[/color][/b]: [list] [*]Um pouco de teoria dos conjuntos [*]Geometria e álgebra do ensino médio [/list] ([b]Nota[/b]: Esse GeoGebraBook é um produto de uma disciplina da graduação em Matemática oferecida na [i]Hong Kong University of Science and Technology[/i])
Table of Contents
- Euclidean Constructions
- Introduction
- Copy a Line Segment
- Bisect an Angle
- Construct an Equilateral Triangle
- Copy a Triangle
- Copy an Angle
- Construct a Perpendicular Bisector and Midpoint
- Construct a Perpendicular Line
- Construct a Parallel Line
- Construct a Point of Division
- Circumcircle of a Triangle
- Using Euclidean Compass
- Tangents of a Circle
- Inscribe a Circle in a Triangle
- Constructibility
- Introduction
- Addition and Subtraction
- Division
- Multiplication
- Squaring a Rectangle
- Taking Square Root
- Constructible Points and Numbers
- Field
- Field Extensions
- Three Classical Problems
- Introduction
- The Main Theorem
- Doubling a Cube
- Double Mean Proportionals
- The Solution of Menaechmus
- The Solution of Erathostenes
- Trisecting an Angle
- Which angle can't be trisected?
- Archimedes' Method of Angle Trisection
- Squaring a Circle
- Theorem of Lindemann
- Quadratrix of Hippias
- Compass-only Constructions
- Straightedge-only Constructions
- Regular Polygons
Introduction
Straightedge
[color=#0000ff][b]Straightedge[/b] [/color] can be regarded as a ruler without any marking.[br][br]The following are exactly what a straightedge can do:[br][list][br][*] Draw a unique straight line through two distinct points[br][*] Extend a line segment arbitrarily far in both direction[br][/list][br]Notice that it [b]cannot[/b] be used for measuring length.
Compass
[color=#0000ff][b]Compass[/b][/color] is a V-shaped drawing tool with a sharp point on one arm and a pencil on another arm. The two arms are joined by a hinge so that the openings of the arms is adjustible.[br][br]Obviously, a compass is mainly used for constructing a circle centered at any given point with any given radius.[br][br]There are two types of compasses:[br][list][br][*] [b]Modern compass[/b] - We can keep the opening fixed when the compass leaves the plane and carry to another location for construction.[br][*] [b]Euclidean (or collapsible) compass[/b] - The compass "forgets" the width of the opening when the compass leaves the plane i.e. we cannot keep the opening fixed.[br][/list]
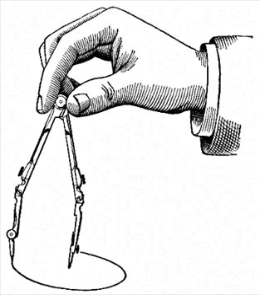
Euclidean Constructions
[color=#0000ff][b]Euclid of Alexandria[/b][/color] (around 300 BC) was a Greek mathematician, who wrote the monumental 13-volume treatise on geometry called "[b]The Elements (幾何原本)[/b]". It laid the foundation of geometry and has been widely considered as the most influential textbook ever written.[br][br]All geometric constructions in "The Elements" only involves straightedge and Euclidean compass. Therefore, such constructions are usually called [b][color=#0000ff]Euclidean constructions[/color][/b].[br][br]There are quite a number of important propositions in "The Elements" which are actually Euclidean constructions. Let us list a few of them here:[br][br][list][*][u]Proposition 1 in Book [/u]I - Construct an equilateral triangle having a given segment as one side.[br][/*][*][u]Proposition 9 in Book I[/u] - Construct the angle bisector of a given angle.[/*][*][u]Proposition 1 in Book III[/u] - Given three non-collinear points, construct the center of the circle containing the three points.[br][/*][*][u]Proposition 11 in Book IV[/u] - Inscribe a regular pentagon (5-sided polygon) in a given circle.[br][/*][/list] [br][br]In this chapter, you will be guided to do various constructions using straightedge and compass. For simplicity, you are allowed to use modern compass instead of the more restrictive Euclidean compass.[br][br]A natural question to think about: Is a modern compass really more powerful than a Euclidean compass? In other words, does there exist something that can be constructed by straightedge and modern compass but not by straightedge and Euclidean compass? We will answer this question later in this chapter.
Introduction
In this chapter, we are going to investigate the following fundamental problem: [br][br][br][i][b][center]What exactly can be constructed by straightedge and compass only?[/center][/b][/i] [br][br]Very generally speaking, we attack this problem by showing that the set of lengths (of line segments) that can be constructed by straightedge and compass only and their negative counterparts, which is usually called the set of [b][color=#0000ff]constructible numbers[/color][/b], possesses a very nice [b][color=#0000ff]algebraic structure[/color][/b] so that we can obtain an elegant criterion to check whether a real number belongs to the above set.
Any positive real number can be expressed as the length of a line segment. Therefore, it makes sense to do arithmetic in a geometric way. We will briefly go through how addition, subtraction, multiplication, division and taking square root can be done by Euclidean constructions. Also, by a combination of these operations, we can produce many more line segments of different lengths in terms of the given ones.
Introduction
After learning knowledge about constructible numbers in the last chapter, we are now ready to tackle the so-called "[b][color=#0000ff]three classical problems[/color][/b]" in ancient Greek geometry. They are[br][br][list][*][color=#0000ff][b][/b][b]Doubling a cube[/b][/color][/*][*][color=#0000ff][b][/b][b]Trisecting an angle[/b][/color][/*][*][color=#0000ff][b][/b][b]Squaring a circle[/b][/color][/*][/list][b][br][/b]These problems were extremely influential in the development of Greek geometry. It turns out that none of the above can be done by Euclidean constructions. We will use the "[b][color=#0000ff]main theorem[/color][/b]" that will be introduced in the next page to prove that it is impossible to double a cube or trisect an angle by straightedge and compass only. As for squaring a circle, a more advanced theorem will be needed.