Realitat Augmentada: Idees per l'Exploració dels Alumnes

Tim Brzezinski, Martha Ivón Cárdenas, Oct 24, 2018

Aquest llibre de GeoGebra conté algunes idees didàctiques per a l'aprenentatge actiu i està enfocat a l'estudiant dins de l'àmbit del GeoGebra amb Realitat Augmentada (AR). Aquest volumen continuarà creixent al llarg del temps.

Table of Contents

  1. Ens iniciem en el GeoGebra amb AR
    1. Ens iniciem en el GeoGebra amb Realitat Augmentada
  2. Idees per a Activitats i Exploració amb els Alumnes
    1. Exploracions de les Seccions Còniques
    2. Rotació sobre l'eix X: Creació de Superfícies de Revolución amb GGB AR
    3. Rotació sobre l'eix Y: Creació de Superfícies de Revolució amb GGB AR
    4. How to Create Surfaces of Revolution in GeoGebra Augmented Reality
  3. Exemples & Demos: Modelat amb AR
    1. GeoGebra Realitat Augmentada: Modelem una Canya (Cilindre)
    2. Modelant una Patata Pringles amb GeoGebra AR
    3. GeoGebra Augmented Reality: Modeling a 2-Piece Lampshade
    4. GeoGebra Augmented Reality: Modeling a Bagel
    5. GeoGebra Augmented Reality: Egg Modeling
    6. Hershey Kiss Modeling in GeoGebra Augmented Reality
    7. GeoGebra Augmented Reality: Building Cookie Models!
    8. Modeling a Fire Hydrant in GeoGebra Augmented Reality
    9. Modeling a Faucet in GeoGebra Augmented Reality
    10. Modeling a 1-Piece + 2-Piece Candle Holder in GeoGebra Augmented Reality
    11. GeoGebra Augmented Reality: STEP SURFACE!
    12. GeoGebra Augmented Reality: Modeling Pottery and Plastic Cups
    13. Modeling a Lampshade (with Parabolic Cross Section) in GeoGebra Augmented Reality
    14. GeoGebra Augmented Reality: Modeling a Bowling Pin
    15. GeoGebra Augmented Reality: Modeling Part of a Ceiling Light Fixture
    16. Modeling a Cone-Head Puppet and Elephant-Shaped Humidifier in GeoGebra Augmented Reality
    17. GeoGebra Augmented Reality: Modeling a Fish
    18. Modeling a Triangular Prism-Shaped Speaker in GeoGebra Augmented Reality
    19. GeoGebra Augmented Reality: Modeling a Plastic Bowl
    20. Modeling a Trampoline + Net in GeoGebra Augmented Reality
    21. Modeling a Charcoal Grill in GeoGebra Augmented Reality
    22. Modeling a Toddler Plate and Frisbee in GeoGebra Augmented Reality
    23. Modeling a Light Bulb in GeoGebra Augmented Reality
    24. Modeling the Side of a Swing in GeoGebra Augmented Reality
    25. Modeling a 2-Piece Light Fixture in GeoGebra Augmented Reality
    26. Modeling a Play Tent in GeoGebra Augmented Reality
    27. Salt Shaker Modeling in GeoGebra Augmented Reality
    28. Flying Fish and 4-Headed Monster Creations in GeoGebra Augmented Reality
  4. AR Modeling Challenges
    1. Toblerone Candy (AR Modeling Challenge)
    2. Square Pyramid with Holes (AR Modeling Challenge)
    3. Square Prism with Holes (AR Modeling Challenge)
    4. Traffic Cone (AR Modeling Challenge)
    5. Random 3D Solid (AR Modeling Challenge)
    6. Lampshade 1 (AR Modeling Challenge)
    7. Deodorant Cap (AR Modeling Challenge)
    8. Trash Can Cover (AR Modeling Challenge)
    9. Headphones Case (AR Modeling Challenge)
    10. Popcorn Bowl (AR Modeling Challenge)
    11. Igloo (AR Modeling Challenge)
    12. Restaurant Light (AR Modeling Challenge)
    13. Lampshade 2 (AR Modeling Challenge)
    14. Bowling Ball Reconstruction (AR Modeling Challenge)
    15. Mailbox Top (AR Modeling Challenge)
    16. Reading Lampshade (AR Modeling Challenge)
    17. Muffin Tin with Muffin (AR Modeling Challenge)
    18. Light Fixture (AR Modeling Challenge)
    19. Wiffle Ball (AR Modeling Challenge)
    20. Ice Cream Cone Head (AR Modeling Challenge)
  5. AR Modeling Tips & Hints
    1. How Domain Restrictions Can Help Students Model in GeoGebra Augmented Reality
    2. Hint for Some GeoGebra AR Modeling Challenges
    3. Quick AR Illustration: Projection of a Surface onto the xy-Plane
  6. Quick Illustrations
    1. Modeling with GeoGebra Augmented Reality: Project Ideas
    2. Basic solids
    3. Penrose triangle
    4. Sierpinski pyramid
    5. Football
    6. Klein Bottle
    7. Ruled Surface
  7. Jornades ACG 2019
    1. AR GeoGebra: passos previs -Android/iOS
    2. AR GeoGebra: Activitats Android
  8. Jornades ACG 2020
    1. Mathcitymap

1.

Ens iniciem en el GeoGebra amb AR

Benvinguts a la Realitat Augmentada!!!

  • 1. Ens iniciem en el GeoGebra amb Realitat Augmentada

1.1.

Ens iniciem en el GeoGebra amb Realitat Augmentada

Demo Ràpida
Tasques:
1) Obre l'App [b][color=#1e84cc]GeoGebra Augmented Reality[/color][/b] en l'iPad o l'iPhone. [br][br]2) Ves a MENU. Selecciona "[b]Two Functions[/b]". [br][br]3) Esborra la primera equació (proporcionada). Per a l'equació blava (superior), escriu [math]z=\sqrt{9-x^2-y^2}[/math]. Per a l'equació inferior, escriu [math]z=-\sqrt{9-x^2-y^2}[/math]. [br][br]4) Agafa una pilota (esfera) i mostra com representa un model perfecte d'esfera. [color=#ff0000]Veure vídeo 0:12 - 0:15.[/color] [br][br]5) Esborra l'equació inferior (rosa). Canvia l'equació blava per [math]z=\sqrt{4x^2+4y^2}[/math]. (Veure minut 0:30 en el vídeo). Es genera un con. [color=#ff0000]Si tens un embut, pots mostrar el que surt al vídeo de 0:44 a 0:54. [/color][br][br]6) Escriu una nova equació rosa z = 3. Com a resultat es mostrarà una de les quatre seccions còniques fonamentals: EL CERCLE. [color=#ff0000]Veure vídeo de 1:02 a 1:13.[/color] [br][br]7) Ara canvia l'equació rosa a z = 2x + 1. Com a resultat es mostrarà una secció transversal (intersecció) que és una PARÀBOLA (2n tipus de secció cònica). [color=#ff0000]Veure vídeo de 1:16 a 1:31. [br][/color][br]8) Ara canvia l'equació rosa a z = 0.5x + 3. Com a resultat es mostrarà una secció transversal (intersecció) que és una EL·LIPSE (3r tipus de secció cònica). Veure vídeo[color=#ff0000] de 1:32 a 1:44. [/color][br][br]9) Ara canvia l'equació rosa a z = 5x + 2. Com a resultat es mostrarà una secció transversal (intersecció) que és una HIPÈRBOLA (4t tipus de secció cònica). Veure vídeo[color=#ff0000] de [/color][color=#ff0000]1:45 al FINAL. [/color][br][br]
[b][color=#1e84cc][url=https://itunes.apple.com/app/geogebra-augmented-reality/id1276964610&utm_source=Download+page&utm_medium=Website&utm_campaign=GeoGebra+Augmented+Reality+for+iOS]Enllaç a GeoGebra Augmented Reality app de l'App Store[/url][/color][/b]
– Martha Ivón Cárdenas, Tim Brzezinski

2.

Idees per a Activitats i Exploració amb els Alumnes

Nou en GeoGebra AR? Fes una ullada a aquestes idees i exploracions didàctiques!!!

  • 1. Exploracions de les Seccions Còniques
  • 2. Rotació sobre l'eix X: Creació de Superfícies de Revolución amb GGB AR
  • 3. Rotació sobre l'eix Y: Creació de Superfícies de Revolució amb GGB AR
  • 4. How to Create Surfaces of Revolution in GeoGebra Augmented Reality

2.1.

Exploracions de les Seccions Còniques

Abans que explorem els tipus de seccions còniques en GeoGebra AR, els explorarem primer amb la Calculadora Gràfica GeoGebra 3D.
1.
[b][color=#ff00ff]Fixa't-hi que l'equació del pla z = alguna constant. [br][br][/color][/b][color=#ff00ff]Canvia l'equació del pla rosa a z = 2. Ara canvia'l a z = 1. Ara canvia'l a z = 4. [/color][br][br]Com descriuries la intersecció entre aquest [b][color=#ff00ff]pla[/color][/b] i el [b][color=#1e84cc]con[/color][/b]?
2.
Canvia l'[b][color=#ff00ff]equació del pla[/color][/b] a [math]z=x+1[/math]. Com descriuries ara la intersecció entre aquest [b][color=#ff00ff]pla[/color][/b] i el [b][color=#1e84cc]con[/color][/b]?
3.
Ara canvia l'[b]equació del pla [/b]a [math]z=0.5x+2.5[/math]. Com descriuries ara la intersecció entre aquest [b][color=#ff00ff]pla[/color][/b] i el [b][color=#1e84cc]con[/color][/b]?
4.
Canvia l'[b][color=#ff00ff]equació del pla[/color][/b] a [math]z=3x+1.5[/math]. Com descriuries ara la intersecció entre aquest [b][color=#ff00ff]pla[/color][/b] i el [b][color=#1e84cc]con[/color][/b]?
Ara explorem aquestes seccions còniques en GGB AR! (Silent "how-to" screencast)
Més: Breu Resum dels Tipus de Seccions Còniques i la seva construcció
– Martha Ivón Cárdenas, Tim Brzezinski

2.2.

–

2.3.

–

2.4.

–

3.

Exemples & Demos: Modelat amb AR

Amb GeoGebra AR, les possibilitats semblen INFINITES! Aquí teniu una petita mostra d'objectes de la vida quotidiana en 3D els quals es poden modelar amb GeoGebra AR. Gaudiu!

  • 1. GeoGebra Realitat Augmentada: Modelem una Canya (Cilindre)
  • 2. Modelant una Patata Pringles amb GeoGebra AR
  • 3. GeoGebra Augmented Reality: Modeling a 2-Piece Lampshade
  • 4. GeoGebra Augmented Reality: Modeling a Bagel
  • 5. GeoGebra Augmented Reality: Egg Modeling
  • 6. Hershey Kiss Modeling in GeoGebra Augmented Reality
  • 7. GeoGebra Augmented Reality: Building Cookie Models!
  • 8. Modeling a Fire Hydrant in GeoGebra Augmented Reality
  • 9. Modeling a Faucet in GeoGebra Augmented Reality
  • 10. Modeling a 1-Piece + 2-Piece Candle Holder in GeoGebra Augmented Reality
  • 11. GeoGebra Augmented Reality: STEP SURFACE!
  • 12. GeoGebra Augmented Reality: Modeling Pottery and Plastic Cups
  • 13. Modeling a Lampshade (with Parabolic Cross Section) in GeoGebra Augmented Reality
  • 14. GeoGebra Augmented Reality: Modeling a Bowling Pin
  • 15. GeoGebra Augmented Reality: Modeling Part of a Ceiling Light Fixture
  • 16. Modeling a Cone-Head Puppet and Elephant-Shaped Humidifier in GeoGebra Augmented Reality
  • 17. GeoGebra Augmented Reality: Modeling a Fish
  • 18. Modeling a Triangular Prism-Shaped Speaker in GeoGebra Augmented Reality
  • 19. GeoGebra Augmented Reality: Modeling a Plastic Bowl
  • 20. Modeling a Trampoline + Net in GeoGebra Augmented Reality
  • 21. Modeling a Charcoal Grill in GeoGebra Augmented Reality
  • 22. Modeling a Toddler Plate and Frisbee in GeoGebra Augmented Reality
  • 23. Modeling a Light Bulb in GeoGebra Augmented Reality
  • 24. Modeling the Side of a Swing in GeoGebra Augmented Reality
  • 25. Modeling a 2-Piece Light Fixture in GeoGebra Augmented Reality
  • 26. Modeling a Play Tent in GeoGebra Augmented Reality
  • 27. Salt Shaker Modeling in GeoGebra Augmented Reality
  • 28. Flying Fish and 4-Headed Monster Creations in GeoGebra Augmented Reality

3.1.

GeoGebra Realitat Augmentada: Modelem una Canya (Cilindre)

Demostració ràpida
– Martha Ivón Cárdenas, Tim Brzezinski

3.2.

–

3.3.

–

3.4.

–

3.5.

–

3.6.

–

3.7.

–

3.8.

–

3.9.

–

3.10.

–

3.11.

–

3.12.

–

3.13.

–

3.14.

–

3.15.

–

3.16.

–

3.17.

–

3.18.

–

3.19.

–

3.20.

–

3.21.

–

3.22.

–

3.23.

–

3.24.

–

3.25.

–

3.26.

–

3.27.

–

3.28.

–

4.

AR Modeling Challenges

This chapter contains 3D modeling challenges for students! For each exercise, the goal for students is to author 2 surface equations (with possible domain restrictions) that models exactly what is seen in a given screencast.

  • 1. Toblerone Candy (AR Modeling Challenge)
  • 2. Square Pyramid with Holes (AR Modeling Challenge)
  • 3. Square Prism with Holes (AR Modeling Challenge)
  • 4. Traffic Cone (AR Modeling Challenge)
  • 5. Random 3D Solid (AR Modeling Challenge)
  • 6. Lampshade 1 (AR Modeling Challenge)
  • 7. Deodorant Cap (AR Modeling Challenge)
  • 8. Trash Can Cover (AR Modeling Challenge)
  • 9. Headphones Case (AR Modeling Challenge)
  • 10. Popcorn Bowl (AR Modeling Challenge)
  • 11. Igloo (AR Modeling Challenge)
  • 12. Restaurant Light (AR Modeling Challenge)
  • 13. Lampshade 2 (AR Modeling Challenge)
  • 14. Bowling Ball Reconstruction (AR Modeling Challenge)
  • 15. Mailbox Top (AR Modeling Challenge)
  • 16. Reading Lampshade (AR Modeling Challenge)
  • 17. Muffin Tin with Muffin (AR Modeling Challenge)
  • 18. Light Fixture (AR Modeling Challenge)
  • 19. Wiffle Ball (AR Modeling Challenge)
  • 20. Ice Cream Cone Head (AR Modeling Challenge)

4.1.

Toblerone Candy (AR Modeling Challenge)

This Toblerone Candy is a triangular prism with bases that are EQUILATERAL TRIANGLES. [br][br]Given that the height of this prism is 6.12 x a base edge, reconstruct what you see here in this screencast in GeoGebra Augmented Reality or in [url=https://www.geogebra.org/3d]GeoGebra's 3D Graphing Calculator[/url]. [br][br][b][color=#1e84cc]Have fun with this! [/color][/b]
– Tim Brzezinski

4.2.

–

4.3.

–

4.4.

–

4.5.

–

4.6.

–

4.7.

–

4.8.

–

4.9.

–

4.10.

–

4.11.

–

4.12.

–

4.13.

–

4.14.

–

4.15.

–

4.16.

–

4.17.

–

4.18.

–

4.19.

–

4.20.

–

5.

AR Modeling Tips & Hints

This chapter contains some hints for students to help complete some of the challenges in the previous chapter (AR Modeling Challenges).

  • 1. How Domain Restrictions Can Help Students Model in GeoGebra Augmented Reality
  • 2. Hint for Some GeoGebra AR Modeling Challenges
  • 3. Quick AR Illustration: Projection of a Surface onto the xy-Plane

5.1.

How Domain Restrictions Can Help Students Model in GeoGebra Augmented Reality

GeoGebra Augmented Reality allows users to input many domain restrictions on multivariable functions! [br][br]Note the equations of the 2 surfaces shown in the screencast below (at bottom of screen). [br][br]The [b][color=#1e84cc]blue surface is a paraboloid of revolution[/color][/b] [math]z=x^2+y^2[/math] for which [math]\left|y\right|\ge1[/math]. [br]The [b][color=#ff00ff]pink surface is part of a parabolic sheet[/color][/b] [math]z=x^2+1[/math] for which [math]\left|x\right|\le1[/math]. [br][br]Put together, these 2 surfaces are used to model the coffee filter shown at the end of this screencast. [br][br]You can enter AS MANY DOMAIN RESTRICTIONS within GeoGebra Augmented Reality as you'd like. Simply put a comma "," after each one.
Quick Demo
– Tim Brzezinski

5.2.

–

5.3.

–

6.

Quick Illustrations

The first resource contains AR photos that Math Teachers have posted on Twitter. The remaining resources (displayed here using GeoGebra's 3D Graphing Calculator) are illustrations of other built-in solids that can be explored within GeoGebra's AR app.

  • 1. Modeling with GeoGebra Augmented Reality: Project Ideas
  • 2. Basic solids
  • 3. Penrose triangle
  • 4. Sierpinski pyramid
  • 5. Football
  • 6. Klein Bottle
  • 7. Ruled Surface

6.1.

Modeling with GeoGebra Augmented Reality: Project Ideas

MATH TEACHERS:
We live in a 3-dimensional world! So why restrict students' creativity to being in the (2D) coordinate plane? [br][br]We can create 3D models of real-world objects within [url=https://www.geogebra.org/3d]GeoGebra's 3D Graphing Calculator.[/url][br]We can also use [b]GeoGebra Augmented Reality[/b] to virtually create & explore such surfaces! [br][br]Below are some photos found on Twitter. We'd love to add YOURS to the list too! [br][br][b][color=#1e84cc]Can you author surface equation(s) (of the form [/color][/b][math]z=[/math][b][color=#1e84cc] )[/color][/b] [b][color=#ff00ff]that model each of the items shown below? [/color][/b] [br][br][b]What other solids exist for which we can create 3D mathematical models? [/b]
Bird Bath (Photo Credit: Jose Luis Munoz)
[url=https://twitter.com/jlmunoz161/status/981103423813832704]Source[/url]
Easter Egg: GREAT Background Sign! (Photo Credit: Owen Elton)
[url=https://twitter.com/owenelton/status/980099069417869314]Source[/url]
Donuts! Hungry yet? (Photo Credit: Steve Phelps)
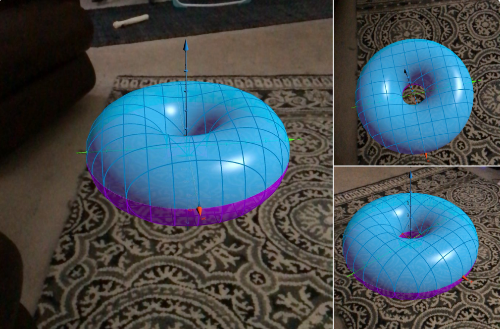
[url=https://twitter.com/giohio/status/1000036148390047744]Source[/url]
Pringles Potato Chip
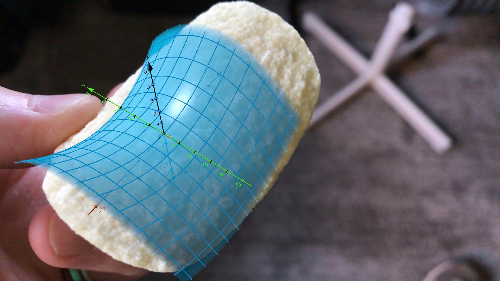
[url=https://twitter.com/dynamic_math/status/987171879143333889]Source[/url]
Conical Cup (Photo Credit: Steve Phelps)
[url=https://twitter.com/giohio/status/994268624721469440]Source[/url]
Toblerone Candy
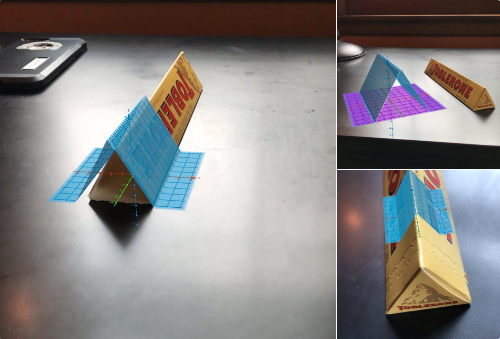
[url=https://twitter.com/dynamic_math/status/994725264956559360]Source[/url]
Roll of Paper Towels (Photo Credit: Steve Phelps)
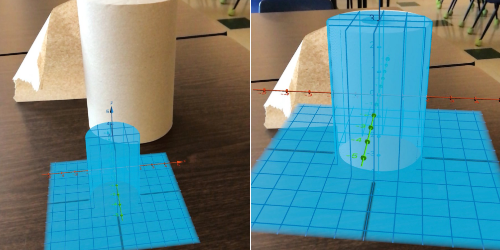
[url=https://twitter.com/giohio/status/994551940225871873]Source[/url]
Stairs (Photo Credit: Steve Phelps)
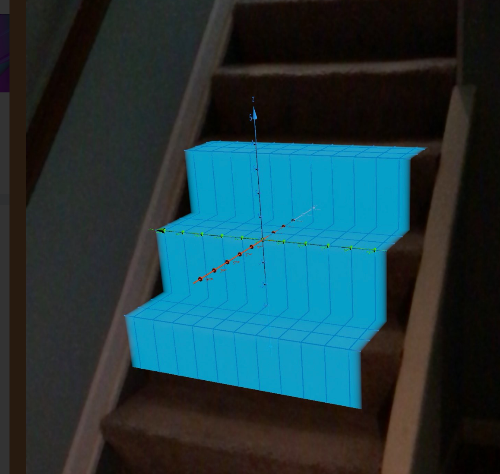
[url=https://twitter.com/giohio/status/1000064066096979970]Source[/url]
Hyperboloid of 1 Sheet Lattice (Photo Credit: Steve Phelps)
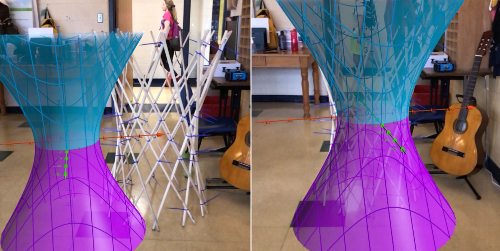
[url=https://twitter.com/giohio/status/994634527904616451]Source[/url]
Basket (Photo Credit: Steve Phelps)
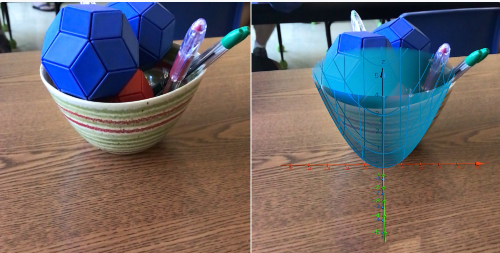
[url=https://twitter.com/giohio/status/994600185438396416]Source[/url]
SUBMIT YOUR GEOGEBRA AUGMENTED REALITY MODELING PHOTOS!
[b]Let's let this list of 3D project modeling ideas GROW! [/b][br][br]Submit your best photo(s) to dynamicmathsolutions@gmail.com [br][br]OR[br][br][b][color=#0000ff]If you're on Twitter,[/color][/b] post them there and be sure to tag [url=https://twitter.com/geogebra]@geogebra[/url], [url=https://twitter.com/dynamic_math]@dynamic_math[/url], #MTBoS, & #ITeachMath!
– Tim Brzezinski

6.2.

–

6.3.

–

6.4.

–

6.5.

–

6.6.

–

6.7.

–

7.

Jornades ACG 2019

  • 1. AR GeoGebra: passos previs -Android/iOS
  • 2. AR GeoGebra: Activitats Android

7.1.

AR GeoGebra: passos previs -Android/iOS

Realitat augmentada amb GeoGebra:[br][br][b]ANDROID/iOS[/b][br][br][u][color=#0000ff][b]INSTAL·LACIONS PRÈVIES[/b][/color][/u][br]1) Instal·lació [b]Android[/b] de AR Core (pas 1):[br][url=https://play.google.com/store/apps/details?id=com.google.ar.core]https://play.google.com/store/apps/details?id=com.google.ar.core[/url][br]2) Instal·lació [b]Android[/b] de GeoGebra 3D (pas 2):[br][url=https://play.google.com/store/apps/details?id=org.geogebra.android.g3d&utm_source=Download+page&utm_medium=Website&utm_campaign=3D+Calculator+for+Android]https://play.google.com/store/apps/details?id=org.geogebra.android.g3d&utm_source=Download+page&utm_medium=Website&utm_campaign=3D+Calculator+for+Android[/url][br]3) Instal·lació [b]iOS[/b] de AR GeoGebra:[br][url=https://itunes.apple.com/app/geogebra-augmented-reality/id1276964610]https://itunes.apple.com/app/geogebra-augmented-reality/id1276964610[/url][br][br][color=#0000ff][b][u]LLISTAT DE MÒBILS I TABLETES COMPATIBLES[/u][/b][/color][br]1) Llista [b]Androids[/b] compatibles:[br][url=https://developers.google.com/ar/discover/supported-devices]https://developers.google.com/ar/discover/supported-devices[/url][br]2) Llista [b]iOS[/b] compatibles:[br][url=https://developers.google.com/ar/discover/supported-devices#ios]https://developers.google.com/ar/discover/supported-devices#ios[br][/url]
– Martha Ivón Cárdenas

7.2.

–

8.

Jornades ACG 2020

Ruta matemàtica amb realitat augmentada

  • 1. Mathcitymap

8.1.

Mathcitymap

Mathcitymap
Com podem crear una ruta matemàtica?
1) Triar el lloc geogràfic.[br]2) Que la ruta tingui un fil conductor: ruta de façanes modernistes, mercats de Barcelona...[br]3) Accedir a les plataformes de creació de rutes.[br] - Mathcitymap: https://mathcitymap.eu/es/[br] - Instamaps: https://www.instamaps.cat/#/[br]4) Afegim realitat augmentada: passos previs[br]https://www.geogebra.org/m/hq3ar8wu
Passeig per Sant Pol amb GeoGebra
– Martha Ivón Cárdenas

Information