Methods of Proving Triangles are congruent
The basic idea is to show that if I give somebody a combination of three components of a triangle, that they would be able to construct a triangle that will fit perfectly on top of my triangle. The question is: What three components can I give them which will always ensure they will be able to do this? Constructions of triangles which are congruent by using minimum requirements allow us to explored this concept. We also need to consider approaches which do not guarantee success.
Table of Contents
- Introduction to congruency
- Exploring Congruency
- Direct construction methods
- Side-Side-Side
- Side-Angle-Side
- Angle-Side-Angle
- Right-angle-Hypotenuse-Side
- Indirect construction method
- Angle-Angle-Side (Or SAA)
- Two problematic methods
- The Angle-Angle-Angle problem (Similarity)
- The "Donkey Theorem", making an ASS of you
- Conclusions
- CPCTC
Exploring Congruency
When triangles are congruent, six facts are always true.
When triangles are congruent, one triangle can be moved (through one, or more, rigid[br]motions) to coincide with the other triangle. All corresponding sides and[br]angles will be congruent.
Consider the following two triangles:
These two triangles are congruent:
[math]\bigtriangleup ABC [/math] [math]\cong\bigtriangleup[/math] KLM[br][br][b]Corresponding sides are congruent:[/b][br]AB [math]\cong[/math] KL[br]BC [math]\cong[/math] LM[br]AC [math]\cong[/math] KM[br][br][b]Corresponding angles are congruent:[/b][br]Angle A [math]\cong[/math] Angle K[br]Angle B [math]\cong[/math] Angle L[br]Angle C [math]\cong[/math] Angle M[br][br]The good news is that when proving triangles congruent, it is not necessary to[br]prove all six facts to show congruency. There are certain ordered combinations[br]of these facts that are sufficient to prove triangles congruent. These[br]combinations guarantee that, given these facts, it will be possible to construct[br]triangles which will take on only one shape (be unique), thus insuring[br]congruency.
Side-Side-Side
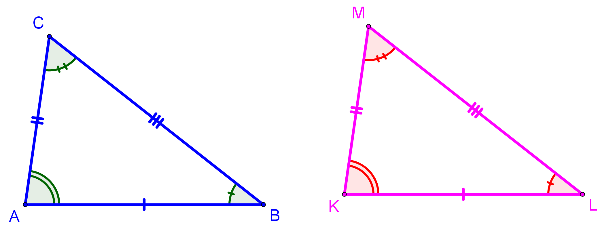
SSS Illustrated
SSS Explained
If three sides of a triangle are congruent to three sides of another triangle, the[br]triangles are congruent.[br][br]Below is an example how to construct this. If you change anything in the construction, just click on the arrows on the top right to restore the construction.
Example:Constructing SSS
Steps in constructing SSS
[center][b][color=#ff0000]Now you try to draw a triangle congruent to the previous one[br][/color][br][color=#38761d]You need to draw a triangle with three sides: AB=5cm, AC=7cm and BC=8 cm. [br]Try to do this in the "Applet" below[br][br][/color][/b][/center][list=1][*]Use [icon]/images/ggb/toolbar/mode_segmentfixed.png[/icon] to draw segment AB and if you are requested to give the length type in 5[br][/*][*]Use [icon]/images/ggb/toolbar/mode_circlepointradius.png[/icon] click on point A and then when requested to provide the radius type in 7[br][/*][*]Use [icon]https://tube.geogebra.org/images/ggb/toolbar/mode_circlepointradius.png[/icon] click on point B and then when requested to provide the radius type in 8[br][/*][*]Use [icon]/images/ggb/toolbar/mode_intersect.png[/icon] to plot point C at the intersection of the two circles[br][/*][*]Use [icon]/images/ggb/toolbar/mode_polygon.png[/icon] and click on point A, B and C to create the triangle[/*][/list]
Your attempt to construct SSS
Exploring SSS
Pythagorean Triples
Read more about Pythagorean Triples by clicking here:[br][br][list][*][url=http://www.mathsisfun.com/pythagorean_triples.html]Pythagorean Triples: Basics[/url][/*][/list][list][*][url=http://www.mathsisfun.com/numbers/pythagorean-triples.html]Pythagorean Triples: Advanced[/url][/*][/list]
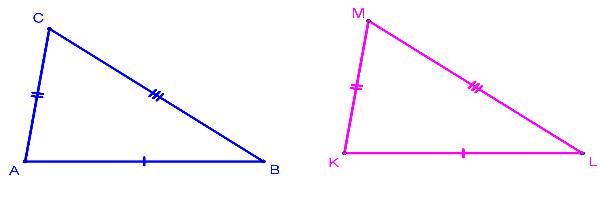
Angle-Angle-Side (Or SAA)
AAS Illustrated
AAS Explained
If two angles and the non-included side of one triangle are congruent to the corresponding parts of another triangle, the triangles are congruent.[br][br][b][color=#0000ff]This is an extension of ASA. In ASA, since you know two sets of angles are congruent, you automatically know the third sets are also congruent since the sum of the interior angles are 180º in each triangle.[/color][/b][br][br]The "non-included" side in AAS can be either of the two sides that are not directly between the two angles being used.[br][br]Below is an example how to construct this. If you change anything in the construction, just click on the arrows on the top right to restore the construction.
Example: Constructing AAS
Steps in constructing AAS
[b][center][color=#ff0000]Now you try to draw a triangle congruent to the previous one[br][/color][br][color=#38761d]You need to draw a triangle with side AB=8cm an angle CAB of 40 degrees and angle BCA of 110 degrees. Try to do this in the "Applet" below[/color][/center][br][/b] [br][list=1][*]Use [icon]https://tube.geogebra.org/images/ggb/toolbar/mode_segmentfixed.png[/icon] to draw segment AB and if you are requested to give the length type in 5[/*][*]Use [icon]https://tube.geogebra.org/images/ggb/toolbar/mode_anglefixed.png[/icon] to draw an angle at point A. ([color=#0000ff][b]Hint: Always click last on the point where you want the angle.[/b][/color]) If requested for the angle size type in 40 degrees. Lastly you need to select clockwise or counterclockwise. The direction of movement is from the line in a clockwise or counterclockwise direction.[/*][*]Use [icon]https://tube.geogebra.org/images/ggb/toolbar/mode_ray.png[/icon] to draw a ray from point A through point B' that were created by the angle tool.[/*][*][color=#0000ff][b]The problem is you cannot draw the next angle as you do not know the length of side AC. But you do know that the sum of the interior angles of a triangle is 180 degrees. This imply that angle ABC=180-(40+110). Now I can construct an angle at point B.[/b][/color][/*][*]Use [icon]https://tube.geogebra.org/images/ggb/toolbar/mode_anglefixed.png[/icon] to draw an angle at point B. If requested for the angle size type in 30 degrees.[/*][*]Use [icon]https://tube.geogebra.org/images/ggb/toolbar/mode_ray.png[/icon] to draw a ray from point B through point A' that were created by the angle tool.[/*][*]Use [icon]https://tube.geogebra.org/images/ggb/toolbar/mode_intersect.png[/icon] to place point C at the intersection of the two rays[br][/*][*]Use [icon]https://tube.geogebra.org/images/ggb/toolbar/mode_polygon.png[/icon] to draw triangle ABC[br][/*][/list]
Exploring AAS
This is the same as for ASA. (See previous lesson)
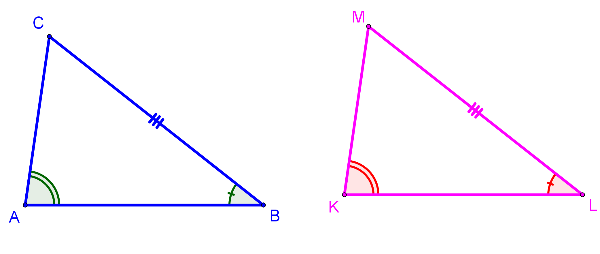
The Angle-Angle-Angle problem (Similarity)
This method will NOT always prove triangles congruent!
Look at the illustration below:
AAA Illustrated
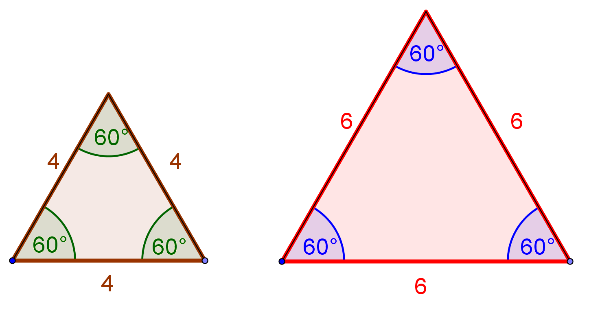
AAA Explained
The AAA combination will show that the triangles are the same SHAPE (similar), but will [b]NOT[/b] show that the triangles are the same size. [i][br][br]Example: [/i]Consider the two equilateral triangles above that satisfy the AAA combination. They are the same shape, but are not the same size. Thus, they are not congruent. (They are similar.)[br][br]Yes,it is possible that the sides "could" be the same length and the[br]triangles would be congruent, but this would be the exception, not the rule.[br][br][b][color=#ff0000]If we prove AAA we are proving similarity and not necessary congruency.[/color][/b][br][br][b]Click here:[color=#0000ff] [/color][/b][url=http://www.sparknotes.com/math/geometry2/congruence/section5.rhtml][color=#0000ff][b]Read more about proving triangles to be similar.[/b][/color][/url]
CPCTC
Corresponding Parts of Congruent Triangles are Congruent
Once triangles are proven congruent, the corresponding leftover "parts" that were not used in SSS, SAS, ASA, AAS and RHS, are also congruent.
Thank you
If you enjoyed using this or you would like to provide me with feedback, please contact me:[br]herholdtb@gmail.com