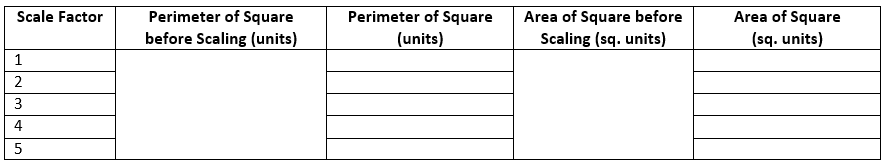
[color=#000000][b][i]CHANGE THE SCALE – WHAT HAPPENS TO AREA AND PERIMETER?[/i][/b][br][br][b]Introduction[br][/b]If we enlarge or shrink a shape, what happens to the resulting area and the perimeter?  In this experiment, you will change the size of a square by adjusting a [i][b]scale factor[/b][/i].  The scale factor determines by how much you want to increase the dimensions of a starting shape – double? …triple? …etc.[br][br][b]Step 1[/b][br]Use the Distance or Length [icon]/images/ggb/toolbar/mode_distance.png[/icon]and Area [icon]/images/ggb/toolbar/mode_area.png[/icon] tools to label the perimeter and area of the green square. [br][br]Use the Move tool [icon]/images/ggb/toolbar/mode_move.png[/icon] to drag the measurement labels to new locations if they get in your way.[br][br][b]Step 2[br][/b]Use the Scale Factor slider [icon]https://tube.geogebra.org/images/ggb/toolbar/mode_slider.png[/icon] to choose how much to enlarge the green square.  [br][br]Complete a Data Table like the one pictured below.[br][br][b]Step 3[/b][br]a)  As the square doubles, triples, etc. in size compared to its original size, what happens to square’s perimeter?[br] [br] [br]b)  As the square doubles, triples, etc. in size compared to its original size, what happens to square’s area?[br] [br] [br] [br][b]Conclusion[/b][br]What is the relationship between scale factor and perimeter?[br] [br] [br] [br]What is the relationship between scale factor and area? [br] [br] [br] [br]If a square is scaled by a factor of 7, explain how you could determine the square’s[br]perimeter and area. [br][br][br][br]Is this just unique to squares?  Experiment with other polygons to find out.[br][br][/color]

[color=#000000][b]Introduction[br][/b]What makes a triangle a triangle?  [br]What makes a triangle a unique triangle – one of a kind?  [br]It’s all about sides and angles.  [br][br]In this activity you will explore the sides of a triangle, noticing when a set of sides, line segments, can determine a unique triangle, more than one triangle, or no triangle at all.[br][br]In the [i]GeoGebra [/i]workspace, there are three sets of sides that can be used to attempt to construct triangles in the open workspace: Set A, Set B, and Set B.[br][br]The red line segments are of fixed length.  [br]The black line segment has an adjustable length, changed by dragging the black endpoint.  [br]They can be moved about the workspace using the blue endpoint.  [br]They can be swiveled using the orange endpoint.[br][br][b]Step 1[br][/b]Use the MOVE tool to drag and manipulate line segments in Set A onto the open workspace.  Try to make a triangle with the given segments as sides. Connect the end-points are carefully as you can. Can you construct a triangle with these three line segments?  Explain your answer.[br][br][b]Step 2[br][/b]Move the line segments from Set A back into their box. Use the MOVE tool to drag and manipulate line segments in Set B onto the open workspace.  Try to make a triangle with the given segments as sides. Connect the end-points are carefully as you can. Can you make a triangle with these three line segments?  Can you make more than one? [br][br][b]Step 3[/b][br]Move the line segments from Set B back into their box. Use the MOVE tool to drag and manipulate line segments in Set C onto the open workspace.  [br][br]Try to make a triangle with the given segments as sides.[br]Can you make a triangle with these three line segments?  [br]Can you make more than one? How many?[br][br][b]Conclusion[/b][br]Summarize what you have learned about the sides of triangles and whether or not, given a set of sides, you can create triangle, more than one triangle, or no triangle at all.[/color]

[color=#000000]Just as a line is made of an infinite number of points, a plane is made of an infinite number of lines that are right next to each other. A plane is flat, and it goes on infinitely in all directions. A sheet of paper represents a small part of one plane. But actually a sheet of paper is much thicker than a plane, because a plane has no thickness. It is only as thick as a point, which takes up no space at all. So a plane is like an imaginary sheet of paper, infinitely wide and long, but with no thickness. [br][br]When we talk about a triangle or a square, these shapes are like pieces cut out of a plane, as if you had cut them out of a piece of paper.  But is there another way to create these polygons?[br][br]Some geometers are very interested what happens when a plane intersects or cuts a 3-Dimensional shape.[br][br]Examine the [i]GeoGebra[/i] workspace.[br][br]The light blue-green rectangle represents, like a piece of paper, a small part of a plane.  [br][br]The dark green shape represents the polygon that would be formed if the plane actually cut the triangular pyramid.[br][br]You can use the slider to MOVE THE PLANE forwards and backwards.  [br]The ROTATE THE VIEW point can be used to look at the pyramid from different angles.[br][br]Experiment with these tools.  Observe what polygons are created when a plane slices through a triangular pyramid.[br][br]What shapes did you observe?[/color]

So why does the area of a circle equal pi times the radius times the radius?[br][br]Area = pi X r X r[br]Area = pi X r[sup]2[/sup][br][br]Experiment with this [i]GeoGebra [/i]applet to find out.