Ch. 1 Review GeoGeBook

Robert Sharpe, Oct 9, 2018

This GeoGeBook is to help you reacquaint yourself with the concepts from the second part of Chapter 1.

Table of Contents

  1. Inductive Reasoning
    1. Fibonacci Sequence
    2. Inductive Reasoning With Data
  2. Conditional Statements
    1. Conditional Statements
  3. Deductive Reasoning
    1. Vertical Angles Theorem
    2. Vertical Angles and Linear Pairs
  4. Writing Proofs
    1. Proving Angles Congruent

1.

Inductive Reasoning

  • 1. Fibonacci Sequence
  • 2. Inductive Reasoning With Data

1.1.

Fibonacci Sequence

The Length of Each Side of Each Square
Find the side length of each square.
The Side Lengths
What is the side length of the smallest square? There are two of them.
What is the side length of the next smallest square?
What is the side length of the next smallest square?
What is the side length of the next smallest square?
What is the side length of the next smallest square?
What is the side length of the next smallest square?
What is the side length of the next smallest square?
If there was another square, what would its side length be?
If there was another square, what would its side length be?
– Robert Sharpe

1.2.

–

2.

Conditional Statements

  • 1. Conditional Statements

2.1.

Conditional Statements

Conditional Statement Practice
For the following conditional statement write it's converse, inverse and contrapositive and state the truth value for each.
Converse
What is the converse to the following conditional statement? [br][br]If there is lightning, then practice is canceled.
Is it True or False?
What is the truth value for the converse?
Inverse
What is the inverse of the conditional statement?[br][br]If two lines are parallel, then the alternate interior angles are congruent.
Contrapositive
What is the contrapositive of the conditional statement?[br][br]If the sum of the angles of a polygon is 540 degrees, then it is a pentagon.
– Robert Sharpe

3.

Deductive Reasoning

  • 1. Vertical Angles Theorem
  • 2. Vertical Angles and Linear Pairs

3.1.

Vertical Angles Theorem

[b]Definition:[/b] [b][color=#b20ea8]Vertical Angles[/color][/b] are angles whose sides form 2 pairs of opposite rays. [br][br]When 2 lines intersect, 2 pairs of vertical angles are formed. [color=#b20ea8]One pair of vertical angles is shown below. [/color] [br][color=#888](Click the other checkbox on the right to display the other pair of vertical angles.) [/color][br][br]Interact with the following applet for a few minutes, then answer the questions that follow.
Directions & Questions: [br][br]1) Complete the following statement (based upon your observations). [br] [br] [color=#b20ea8][b]Vertical angles are always __________________________.[/b] [/color] [br][br]2) Suppose the pink angle above measures 140 degrees. What would the measure of its vertical angle? What would be the measure of the other 2 (gray) angles?
– Tim Brzezinski

3.2.

–

4.

Writing Proofs

  • 1. Proving Angles Congruent

4.1.

Proving Angles Congruent

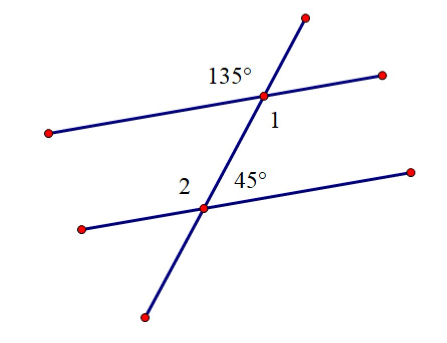
Prove the following:
Prove: [math]\angle1\cong\angle2[/math][br]Given: The measurements of the two angles
What theorem helps you know the measure of angle 1?
What theorem helps you know the measure of angle 2?
On a piece of paper, complete the proof that shows the two angles congruent.
– Robert Sharpe

Information