Trigonometry by S N Attar

Tim Brzezinski, Shamashuddin Attar, Oct 7, 2018

Accompanies MATH 115: Trigonometry (S N ATTAR SHIRGAON)

Table of Contents

  1. Preliminary Content
    1. Perpendicular Lines: Quick Intro
    2. Complementary Meaning?
    3. Supplementary Angles (Quick Exploration)
    4. Isosceles Triangle (Quick Construction Technique) V1
    5. Equilateral Triangle Construction (Dynamic Illustration)
    6. Proof Without Words
    7. Proof Without Words
    8. Practice: Pythagorean Theorem (1)
    9. Quiz: Pythagorean Theorem (2B)
    10. Simplifying Radicals (I)
    11. Simplifying Radicals (II)
    12. Animation 171
    13. Isosceles Right Triangle: Quick Investigation
    14. Another Special Right Triangle (Guided Discovery)
    15. Special Right Triangle (II)
    16. Quiz: Special Right Triangles
    17. Vertical Angles Exploration (1)
    18. Naming Angle Positions
    19. Transversal Intersects Parallel Lines
    20. Corresponding Angles: Quick Investigation
    21. Alternate Interior Angles: Quick Investigation
    22. Alternate Exterior Angles: Quick Investigation
    23. Same-Side-Interior Angles: Quick Investigation
    24. Same-Side-Exterior Angles: Quick Investigation
    25. Similar Figures: Dynamic Illustration
    26. AA Similarity Theorem
    27. SSS ~ Theorem (V2)
  2. Angles in Standard Position (and Related Vocabulary)
    1. Angles in Standard Position
    2. Angle Orientations (Standard Position)
    3. Coterminal Angles Action!!!
  3. The 6 Trigonometric Ratios; Right Triangle Trigonometry; Applications
    1. Quiz: Evaluating Trigonometric Functions of Angles Given a Point on its Terminal Ray
    2. Evaluating Trig Functions Given a Point on the Terminal Ray
    3. Quiz: Quadrant Logic (Trig)
    4. Trig Ratio Possibilities (Hints)
    5. Quiz: Evaluating Trig FNS of Angles (Quadrant Logic): V1
    6. Quiz: Evaluating Trig FNS of Angles WITH Quadrant Logic (V2)
    7. Right Triangle Trigonometry: Intro
    8. True Meaning of Sine, Cosine, Tangent Ratios within Right Triangles
    9. Trig Ratio Estimations (Right Triangle Context)
    10. Trig Ratio Estimations (Quiz: V1)
    11. Right Triangle Trig: Solving for Sides (2)
    12. Quiz: Expressing Angles Using Inverse Trigonometric Function
    13. What "CO" in COsine Means
  4. Introduction to Radians; Arc Length and Area of a Sector
    1. Circle vs. Sphere
    2. Circle Terminology
    3. TRUE MEANING of π
    4. Circumference = ? (Animation)
    5. Circumference = ? (Animation II)
    6. 1 Radian: Clear Definition
    7. Radian Illustrator
    8. DEG to RAD and RAD to DEG Illustrator
    9. DEG to RAD and RAD to DEG: Question Generator
    10. Arc length: Quick Investigation
    11. Parallelogram: Area
    12. Triangle Area Action!!! (V2)
    13. Circle Area (By Peeling!)
    14. Arc Length and Area of a Sector (V1)
    15. Arc Length and Area of a Sector (V2)
    16. Area of a Sector
    17. Angular to Linear: Without Words
    18. YOUR Linear Speed?
  5. Trigonometric Functions of Special Angles
    1. Trig Function Values (30 Degrees)
    2. Trig Function Values (45 Degrees)
    3. Trig Function Values (60 Degrees)
    4. Special Angles in Standard Position: Intro (V2)
    5. Special Angles in Standard Position: Intro (V3)
    6. Quiz 1: Trigonometric Functions of Special Angles
    7. Quiz 2: Trigonometric Functions of Special Angles
  6. Graphing and Writing Trigonometric Functions
    1. Unit Circle and Sine Graph
    2. Unit Circle and Cosine Graph
    3. Transforming Sine and Cosine Functions (1): Dynamic Illustrator
    4. Transforming Sine and Cosine Functions (2): Dynamic Illustrator
    5. Quiz: Graphing Sine & Cosine Functions (Amplitude & Vertical Shift Only)
    6. Writing Sine and Cosine Functions (with Amplitude & Vertical Shift Changes)
    7. Periodic Function Action!
    8. Sine & Cosine Period Action (1)!
    9. Graphing Sine & Cosine Functions (I)
    10. Graphing Sine & Cosine Functions (II)
    11. Modeling with Trigonometric Functions
  7. Advanced Topics
    1. Special Trigonometric Identities: Quick Exploration
    2. Law of Sines (& Area)
    3. Law of Cosines: Discovery
  8. Bearings (Applications)
    1. Bearing Illustrator (1)
    2. Bearing: Dynamic Illustration
    3. Bearing Illustrator (2)
    4. Bearings: Dynamic Illustrator
  9. Vectors
    1. Vectors: Magnitude & Direction (I)
    2. Scaling Vectors
    3. Vector Addition
    4. Vector Subtraction

1.

Preliminary Content

  • 1. Perpendicular Lines: Quick Intro
  • 2. Complementary Meaning?
  • 3. Supplementary Angles (Quick Exploration)
  • 4. Isosceles Triangle (Quick Construction Technique) V1
  • 5. Equilateral Triangle Construction (Dynamic Illustration)
  • 6. Proof Without Words
  • 7. Proof Without Words
  • 8. Practice: Pythagorean Theorem (1)
  • 9. Quiz: Pythagorean Theorem (2B)
  • 10. Simplifying Radicals (I)
  • 11. Simplifying Radicals (II)
  • 12. Animation 171
  • 13. Isosceles Right Triangle: Quick Investigation
  • 14. Another Special Right Triangle (Guided Discovery)
  • 15. Special Right Triangle (II)
  • 16. Quiz: Special Right Triangles
  • 17. Vertical Angles Exploration (1)
  • 18. Naming Angle Positions
  • 19. Transversal Intersects Parallel Lines
  • 20. Corresponding Angles: Quick Investigation
  • 21. Alternate Interior Angles: Quick Investigation
  • 22. Alternate Exterior Angles: Quick Investigation
  • 23. Same-Side-Interior Angles: Quick Investigation
  • 24. Same-Side-Exterior Angles: Quick Investigation
  • 25. Similar Figures: Dynamic Illustration
  • 26. AA Similarity Theorem
  • 27. SSS ~ Theorem (V2)

1.1.

Perpendicular Lines: Quick Intro

WARM UP:
The lines below are said to be [b]perpendicular lines[/b]. [br][br]
Move the LARGE POINTS around a bit, then answer the question that appears below.
[b]What does it mean for two lines to be perpendicular? [/b]
– Tim Brzezinski

1.2.

–

1.3.

–

1.4.

–

1.5.

–

1.6.

–

1.7.

–

1.8.

–

1.9.

–

1.10.

–

1.11.

–

1.12.

–

1.13.

–

1.14.

–

1.15.

–

1.16.

–

1.17.

–

1.18.

–

1.19.

–

1.20.

–

1.21.

–

1.22.

–

1.23.

–

1.24.

–

1.25.

–

1.26.

–

1.27.

–

2.

Angles in Standard Position (and Related Vocabulary)

  • 1. Angles in Standard Position
  • 2. Angle Orientations (Standard Position)
  • 3. Coterminal Angles Action!!!

2.1.

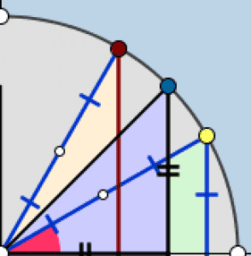
Angles in Standard Position

The angle drawn below in the coordinate plane is classified as being drawn in [b]STANDARD POSITION. [br][br][/b]Interact with the applet for a minute.[br]Then answer the question that follows.
ANGLE IN STANDARD POSITION:
1.
What does it mean for an angle drawn in the coordinate plane to be drawn in [b]STANDARD POSITION? [br][br][/b](Your definition should list 2 criteria.)
– Tim Brzezinski

2.2.

–

2.3.

–

3.

The 6 Trigonometric Ratios; Right Triangle Trigonometry; Applications

  • 1. Quiz: Evaluating Trigonometric Functions of Angles Given a Point on its Terminal Ray
  • 2. Evaluating Trig Functions Given a Point on the Terminal Ray
  • 3. Quiz: Quadrant Logic (Trig)
  • 4. Trig Ratio Possibilities (Hints)
  • 5. Quiz: Evaluating Trig FNS of Angles (Quadrant Logic): V1
  • 6. Quiz: Evaluating Trig FNS of Angles WITH Quadrant Logic (V2)
  • 7. Right Triangle Trigonometry: Intro
  • 8. True Meaning of Sine, Cosine, Tangent Ratios within Right Triangles
  • 9. Trig Ratio Estimations (Right Triangle Context)
  • 10. Trig Ratio Estimations (Quiz: V1)
  • 11. Right Triangle Trig: Solving for Sides (2)
  • 12. Quiz: Expressing Angles Using Inverse Trigonometric Function
  • 13. What "CO" in COsine Means

3.1.

Quiz: Evaluating Trigonometric Functions of Angles Given a Point on its Terminal Ray

– Tim Brzezinski

3.2.

–

3.3.

–

3.4.

–

3.5.

–

3.6.

–

3.7.

–

3.8.

–

3.9.

–

3.10.

–

3.11.

–

3.12.

–

3.13.

–

4.

Introduction to Radians; Arc Length and Area of a Sector

  • 1. Circle vs. Sphere
  • 2. Circle Terminology
  • 3. TRUE MEANING of π
  • 4. Circumference = ? (Animation)
  • 5. Circumference = ? (Animation II)
  • 6. 1 Radian: Clear Definition
  • 7. Radian Illustrator
  • 8. DEG to RAD and RAD to DEG Illustrator
  • 9. DEG to RAD and RAD to DEG: Question Generator
  • 10. Arc length: Quick Investigation
  • 11. Parallelogram: Area
  • 12. Triangle Area Action!!! (V2)
  • 13. Circle Area (By Peeling!)
  • 14. Arc Length and Area of a Sector (V1)
  • 15. Arc Length and Area of a Sector (V2)
  • 16. Area of a Sector
  • 17. Angular to Linear: Without Words
  • 18. YOUR Linear Speed?

4.1.

Circle vs. Sphere

[color=#000000]Interact with the applet below for a few minutes, then answer the questions that follow. Be sure to move the BIG POINTS around during your investigation! [/color]
[color=#980000][b]Questions:[/b][/color][br][br][color=#000000]1) What do a CIRCLE and SPHERE have in common? Explain.[br][br]2) How are a CIRCLE and SPHERE different? Explain. [br][br]3) Using what you've observed, write a definition for the term CIRCLE and for the term SPHERE. [br] [i]Hint: Be sure to use the words "locus", "points", and "equidistant" in each definition! [/i][/color]
– Tim Brzezinski

4.2.

–

4.3.

–

4.4.

–

4.5.

–

4.6.

–

4.7.

–

4.8.

–

4.9.

–

4.10.

–

4.11.

–

4.12.

–

4.13.

–

4.14.

–

4.15.

–

4.16.

–

4.17.

–

4.18.

–

5.

Trigonometric Functions of Special Angles

  • 1. Trig Function Values (30 Degrees)
  • 2. Trig Function Values (45 Degrees)
  • 3. Trig Function Values (60 Degrees)
  • 4. Special Angles in Standard Position: Intro (V2)
  • 5. Special Angles in Standard Position: Intro (V3)
  • 6. Quiz 1: Trigonometric Functions of Special Angles
  • 7. Quiz 2: Trigonometric Functions of Special Angles

5.1.

Trig Function Values (30 Degrees)

Interact with the applet below for a few minutes. Then answer the questions that follow.
LARGE POINTS are MOVEABLE. Be sure to slide the slider (lower right) slowly. As you do, pay careful attention to what you see here.
1.
Just by merely observing the dynamics of this applet, what would the [color=#9900ff][b]sine of 30 degrees [/b][/color]be?
2.
What would the [color=#9900ff][b]cosecant of 30 degrees[/b][/color] be?
3.
Do the values of [color=#9900ff][b]either of these ratios[/b][/color] depend upon [color=#666666][b]the length of the circle's radius[/b][/color]? Explain why or why not.
– Tim Brzezinski

5.2.

–

5.3.

–

5.4.

–

5.5.

–

5.6.

–

5.7.

–

6.

Graphing and Writing Trigonometric Functions

  • 1. Unit Circle and Sine Graph
  • 2. Unit Circle and Cosine Graph
  • 3. Transforming Sine and Cosine Functions (1): Dynamic Illustrator
  • 4. Transforming Sine and Cosine Functions (2): Dynamic Illustrator
  • 5. Quiz: Graphing Sine & Cosine Functions (Amplitude & Vertical Shift Only)
  • 6. Writing Sine and Cosine Functions (with Amplitude & Vertical Shift Changes)
  • 7. Periodic Function Action!
  • 8. Sine & Cosine Period Action (1)!
  • 9. Graphing Sine & Cosine Functions (I)
  • 10. Graphing Sine & Cosine Functions (II)
  • 11. Modeling with Trigonometric Functions

6.1.

Unit Circle and Sine Graph

Anthony Or. GeoGebra Institute of Hong Kong.[br]orchiming@gmail.com
– Anthony OR 柯志明

6.2.

–

6.3.

–

6.4.

–

6.5.

–

6.6.

–

6.7.

–

6.8.

–

6.9.

–

6.10.

–

6.11.

–

7.

Advanced Topics

  • 1. Special Trigonometric Identities: Quick Exploration
  • 2. Law of Sines (& Area)
  • 3. Law of Cosines: Discovery

7.1.

Special Trigonometric Identities: Quick Exploration

1.
Write "?" in terms of [math]\theta[/math]. What do you get?
2.
In terms of the symbols "%", "$", and/or "!", what is [math]sin\left(\theta\right)[/math]?
3.
In terms of the symbols "%", "$", and/or "!", what is [math]cos\left(?\right)[/math]
4.
Take your results from (1) and (3) to write a new trigonometric identity. What do you get?
5.
In terms of the symbols "%", "$", and/or "!", what is [math]cos\left(\theta\right)[/math]?
6.
In terms of the symbols "%", "$", and/or "!", what is [math]sin\left(?\right)[/math]
7.
Take your results from (1) and (6) to write a new trigonometric identity. What do you get?
– Tim Brzezinski

7.2.

–

7.3.

–

8.

Bearings (Applications)

  • 1. Bearing Illustrator (1)
  • 2. Bearing: Dynamic Illustration
  • 3. Bearing Illustrator (2)
  • 4. Bearings: Dynamic Illustrator

8.1.

Bearing Illustrator (1)

In trigonometry, [b][color=#9900ff]a bearing[/color][/b] is a means of describing one point's location with respect to another.[br][br]The applet below illustrates point B's bearing with respect to point [i]A[/i]. [br]Slide the [b][color=#9900ff]BEARING[/color][/b] slider to change the bearing from point [i]A[/i] to point [i]B[/i]. [br]You can also move any of the 3 points around at any time. [br][br]Then, answer the questions that follow. [br][br]
1.
In words, describe what the bearing from point [i]A[/i] to point [i]B [/i]means. Be sure to be detailed in your description!
2.
Can you give another bearing equivalent to a bearing of 120 degrees? Why is this?
3.
If point [i]B[/i] is directly south of [i]A[/i], what is point [i]B[/i]'s bearing from [i]A[/i]?
4.
If point [i]B[/i] is directly west of [i]A[/i], what is point [i]B[/i]'s bearing from [i]A[/i]?
– Tim Brzezinski

8.2.

–

8.3.

–

8.4.

–

9.

Vectors

Applets about Vector Operations

  • 1. Vectors: Magnitude & Direction (I)
  • 2. Scaling Vectors
  • 3. Vector Addition
  • 4. Vector Subtraction

9.1.

Vectors: Magnitude & Direction (I)

[color=#000000]A vector is a mathematical quantity with both magnitude and direction. [br][br]The[b] magnitude of vector u[/b], denoted |[b]u[/b]|, is it's length. [br][color=#a64d79]One way to express its direction is to give the angle it makes with a horizontal ray (that points to the right) that is parallel to the positive x-axis. This is called the vector's [b]direction angle. [/b][/color][b][br][/b][br]See below. You can also change the locations of the vector's initial point and terminal point[/color]. [br][br][color=#0000ff][b]KEY QUESTION:[/b] Given a vector's magnitude and direction, how can you rewrite this vector in component form? < , > ? [/color]
– Tim Brzezinski

9.2.

–

9.3.

–

9.4.

–

Information