Earth and Sun
Earth and Sun
Table of Contents
- Earth
- Earth (basic model)
- Earth's Cities
- Earth (cities, references, curves...)
- Mercator Projection
- Sun
- Earth and Sun (celestial coordinate system)
- Moon and night sky
- Earth, Sun and Moon
- Moon phases
- Armillary Sphere
Earth (basic model)
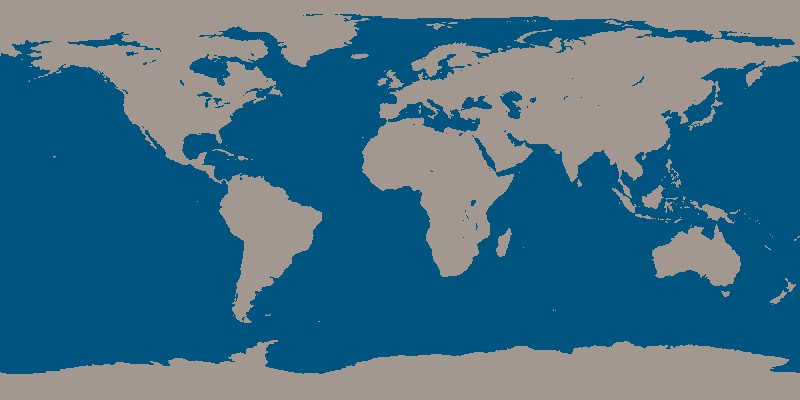
[color=#999999]This activity belongs to the [i]GeoGebra book[/i] [url=https://www.geogebra.org/m/uT3czZnE]Earth and Sun[/url].[/color][br][br][/color]Try wrapping a ball with wrapping paper, and you'll realize that due to its "curvature in all directions," there will always be spaces between the ball and the paper; achieving a perfect fit is impossible.To simplify, let's suppose the shape of the Earth is perfectly spherical. For the most faithful representation of the Earth, it's best to use a globe, because here, just like when wrapping a ball, any attempt to "flatten" the Earth's surface will always result in some kind of distortion, some kind of deviation from the correct measurements (whether distances, angles, or areas).This is because a sphere cannot be "unrolled," meaning its surface cannot be extended onto a plane. The following image corresponds to a world map. We recognize the outlines of the continents, but since it's a flat image rather than a spherical surface, we know it must necessarily be distorted. All world flat maps, in some way, "lie".
In this case, the spherical surface has been "painted" as if the Earth were a cylinder rolling on the paper (this method of projection is called cylindrical projection and, when applied to the Earth, is known as the Mercator projection). Notice that the Arctic (with its ice mass represented as a solid) and Antarctica appear extremely exaggerated, as both the North Pole and the South Pole stretch across the entire length of the image, instead of being represented as points.
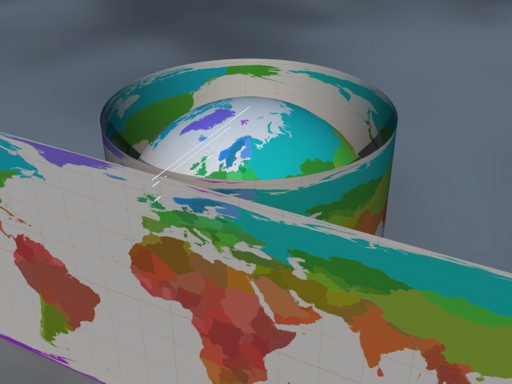
For these reasons, it is advisable to have a globe template rather than relying on a world map, as shown in the following construction.[br][br][color=#999999]For better execution, it's recommended to download de GGB file.[/color]
[color=#999999]Author of the construction of GeoGebra: [color=#999999][url=https://www.geogebra.org/u/rafael]Rafael Losada[/url][/color][/color]
Earth and Sun (celestial coordinate system)
[color=#999999]This activity belongs to the [i]GeoGebra book[/i] [url=https://www.geogebra.org/m/uT3czZnE]Earth and Sun[/url].[/color][br][br]This activity will help you visualize the apparent path of the Sun throughout the year and its consequences, such as the length of the day or the passage of seasons. You can choose from five menus: References, Time, Celestial Coordinates, Coordinates A, and Apparent Trajectory.[br][br][color=#999999]For better execution, and to be able to scale the sphere, it is recommended to [url=https://www.geogebra.org/material/download/format/file/id/mhrhfcfe]download the GGB file[/url].[/color]
[color=#999999]Author of the construction of GeoGebra: [color=#999999][url=https://www.geogebra.org/u/rafael]Rafael Losada[/url][/color][/color]
Earth, Sun and Moon
[color=#999999]Esta actividad pertenece al [i]libro de GeoGebra[/i] [url=https://www.geogebra.org/m/BEUGAvQj]La Tierra y el Sol[/url].[/color][br][br]In this construction, you can observe the positioning, relative to Earth, of the Sun and the Moon. The left panel displays the corresponding phase of the Moon as seen from Earth. Please note that the visualization of this phase depends on the observer's latitude on Earth (latitude of the red point). For example, during the waxing crescent phase, if you center Earth's view on the North Pole, the Moon appears "to the right" of the Sun, but when you center the view on the South Pole, the same Moon appears "to the left" of the Sun.[br][br]Notice that the Moon is not found directly on the ecliptic but rather slightly above or below it. Its orbit, which varies over time, forms an angle of about 5° with the ecliptic and intersects it only at two points, known as [i]nodes[/i]. Solar eclipses [url=https://astronoo.com/images/soleil/grains-de-baily.jpg][img]https://www.geogebra.org/resource/scjbyz2p/0tuzuVw455vxurEw/material-scjbyz2p.png[/img][/url] are only possible when the Moon is at a node (or near it) and is positioned between Earth and the Sun, as during a total solar eclipse, the observer must be aligned with the centers of the Moon and the Sun.
[color=#999999]Author of the construction of GeoGebra: [color=#999999][url=https://www.geogebra.org/u/rafael]Rafael Losada[/url][/color][/color]